Effect and compensate of grating curvature radius error in Seya-Namioka monochromator
-
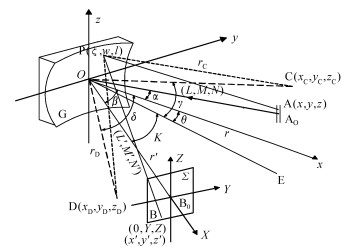
摘要: Seya-Namioka光栅制作过程中的曲率半径误差会引起离焦像差,该像差会对光栅单色仪的光谱性能造成极大的影响。本文基于光线追迹理论,模拟分析了曲率半径误差对Seya-Namioka光栅的具体影响。分析结果表明,出入臂长度对曲率半径误差有很好的补偿作用,通过调整出入臂长度曲率半径误差的容许范围可增大到2 mm左右。总调整量不变的情况下,任意改变出入臂的长度,补偿效果相似。随着误差的增加需要调整的出入臂长度值变大,过大的误差使用出入臂长度无法进行补偿;出入臂夹角仅能对正向曲率半径误差进行补偿,且补偿所需调整角过大,影响单色仪的结构设计,该方法并不实用。结果可为单色仪的设计和使用提供理论参考。
-
关键词:
- 曲率半径误差 /
- Seya-Namioka光栅 /
- 光线追迹 /
- 使用参数 /
- 误差补偿
Abstract: The curvature radius error in the Seya-Namioka grating process can cause defocus aberration, which may have a great impact on the spectral performance of the grating monochromator. Based on the theory of ray tracing, we simulate and analyze the impact of curvature radius error on Seya-Namioka grating. The analysis results show that the length of the entrance and exit arm has a good compensating effect on the curvature radius error. By adjusting the length of the entrance and exit arm, the allowable range of the curvature radius error can be increased to about 2 mm. If the total adjustment amount is not changed and the length of the access arm is arbitrarily changed, the compensation effect is similar. As the error increases, the length of the arm that needs to be adjusted becomes larger, and the excessive error makes the length of the arm unable to be compensated. In addition, changing the angle between the arm and the arm can only compensate for the error of the positive curvature radius. The adjustment angle needed for compensation is too large, which will affect the design structure of the monochromator, and it is not practical to compensate the curvature radius error by changing the angle of the entrance and exit arm. The research results in this paper show that by adjusting the parameters of the grating, the influence of errors can be reduced and the margin of error can be extended.-
Key words:
- curvature radius error /
- Seya-Namioka grating /
- ray-tracing /
- error compensation /
-
表 1 总调整量与曲率半径误差的关系
Table 1. Relationship between the total adjustment amount and radius error
曲率半径误差值/mm 0.1 0.2 0.5 1.0 2.0 总调整量/mm 0.3 0.5 1.3 2.6 5.3 -
[1] 吴国安.光谱仪器设计[M].北京:科学出版社, 1987.WU G A. Design of Spectroscopic Instruments[M]. Beijing:Science Press, 1978.(in Chinese) [2] 卢启鹏, 宋源, 等.极高分辨变包含角平面光栅单色器关键技术及检测方法研究[J].中国光学, 2016, 9(2):281-295. http://www.chineseoptics.net.cn/CN/abstract/abstract9026.shtmlLU Q P, SONG Y, et al.. Key technologies and the performance measuring methods in variable included angle plane grating monochromator[J]. Chinese Optics, 2016, 9(2):281-295.(in Chinese) http://www.chineseoptics.net.cn/CN/abstract/abstract9026.shtml [3] NODA H, NAMIOKA T, SEYA M. Geometrical theory of the grating[J]. J. Opt. Soc. Am, 1974, 64:1031-1036. doi: 10.1364/JOSA.64.001031 [4] 寇婕婷, 吴娜, 等.凹面光栅衍射效率测试仪精度分析与优化[J].光学 精密工程, 2012, 20(6):1225-1232. http://www.cnki.com.cn/Article/CJFDTOTAL-GXXB201509048.htmKOU J T, WU N, et al.. Precision analysis and optimization on diffraction efficiency instrument for concave gratings[J]. Opt. Precision Eng., 2012, 20(6):1225-1232.(in Chinese) http://www.cnki.com.cn/Article/CJFDTOTAL-GXXB201509048.htm [5] 姜岩秀, 韩建, 等.平面全息光栅曝光系统中的分光器件特性分析[J].中国光学, 2015, 8(2):241-247. http://www.chineseoptics.net.cn/CN/abstract/abstract9254.shtmlJANG Y X, HAN J, et al.. Characteristic analysis for different beamsplitters of the plane holographic grating lithography system[J]. Chinese Optics, 2015, 8(2):241-247.(in Chinese) http://www.chineseoptics.net.cn/CN/abstract/abstract9254.shtml [6] NODA H, NAMIOKA T, SEYA M. Design of holographic concave gratings for Seya-Namioka monochromators[J]. J. Opt. Soc. Am, 1974, 64(8):1043-1048. doi: 10.1364/JOSA.64.001043 [7] NAMIOKA T, SEYA M, NODA H. Design and performance of holographic concave gratings[J]. J. Appl. Phys., 1976, 15(7):1181-1197. doi: 10.1143/JJAP.15.1181 [8] MCKINNEY W R, PALMER C. Numerical design method for aberration reduced concave grating spectrometers[J]. Appl. Opt., 1987, 26(15):3108-3118. doi: 10.1364/AO.26.003108 [9] 包仁, 宋从龙.Ⅳ型消像差全息凹面光栅的设计与实验[J].仪器仪表学报, 1988, 9:240-246. http://www.wanfangdata.com.cn/details/detail.do?_type=degree&id=Y1614739BAO R, SONG C L. A design of type Ⅳ aberration-corrected holographic concave grating[J]. Chinese Journal of Scientific Instrument, 1988, 9:240-246.(in Chinese) http://www.wanfangdata.com.cn/details/detail.do?_type=degree&id=Y1614739 [10] SOKOLOVA E A, KRUIZINGA V, BRUIJN D D, et al.. Computer modelling of a wavefront diffracted at a concave grating[J]. Opt. Technol., 2003, 70(8):600-606. doi: 10.1364/JOT.70.000600 [11] ELENA SOKOLOVA. Simulation of mechanically ruled concave diffraction gratings by use of an original geometric theory[J]. Appl. Opt., 2004, 43(1):20-28. doi: 10.1364/AO.43.000020 [12] 王秋平, 余小江, 高辉, 等.光栅单色器及相关技术[J].现代科学仪器, 2001, 1:49-52. http://cpfd.cnki.com.cn/Article/CPFDTOTAL-ZGGY200400001583.htmWANG Q P, YU X J, GAO H, et al.. Grating monochromator related techniques[J]. Modern Scientific Instruments, 2001, 1:49-52.(in Chinese) http://cpfd.cnki.com.cn/Article/CPFDTOTAL-ZGGY200400001583.htm [13] SINGH M, REDDY G P. Seya-Namioka monochromators theory and design of holographic concave gratings[J]. Optik, 1986(74):142-153. https://www.researchgate.net/publication/279768415_SEYA-NAMIOKA_MONOCHROMATORS_THEORY_AND_DESIGN_OF_HOLOGRAPHIC_CONCAVE_GRATING [14] 孔鹏, 唐玉国, 巴音贺希格, 等.平场全息凹面光栅结构参量误差之间的补偿作用[J].光学学报, 2011, 31(7):0705001-6. http://www.irgrid.ac.cn/handle/1471x/599559KONG P, TANG Y G, BAYANHESHIG, et al.. Compensation effects between parameter error of flat-field holographic concave gratings[J]. Acta Optica Sinica, 2011, 31(7):0705001-6.(in Chinese) http://www.irgrid.ac.cn/handle/1471x/599559 [15] 曾瑾, 巴音贺希格, 李文昊, 等.Ⅳ型凹面全息光栅参数误差对光谱性能的影响及补偿[J].光学学报, 2011, 31(10):1005005-7. http://www.cnki.com.cn/Article/CJFDTOTAL-GXXB201110011.htmZENG J, BAYANHESHIG, LI W H, et al.. Effect of type-Ⅳ concave holographic grating parameter errors on spectral performance and their compensation[J]. Acta Optica Sinica, 2011, 31(10):1005005-7.(in Chinese) http://www.cnki.com.cn/Article/CJFDTOTAL-GXXB201110011.htm [16] 周倩, 曾理江, 李立峰.平场全息凹面光栅制作结构与使用结构之间误差补偿作用的数值模拟与实验验证[J].光谱学与光谱分析, 2008, 28(7):1674-1678. http://www.opticsjournal.net/abstract.htm?id=OJ110620000168B9EbHdZHOU Q, ZENG L J, LI L F. Numerical simulation of error compensation between recording structure and use structure of flat-field holographic concave grating[J]. Spectroscopy and Spectral Analysis, 2008, 28(7):1674-1678.(in Chinese) http://www.opticsjournal.net/abstract.htm?id=OJ110620000168B9EbHd [17] 吕丽军, 石亮.平面对称光学系统像差理论的扩展[J].光学 精密工程, 2009, 17(12):2975-2982. doi: 10.3321/j.issn:1004-924X.2009.12.015LV L J, SHI L.Generalization of aberration theory of plane-ssymmetric optical systems[J]. Opt. Precision Eng., 2009, 17(12):2975-2982.(in Chinese) doi: 10.3321/j.issn:1004-924X.2009.12.015 -






 下载:
下载: