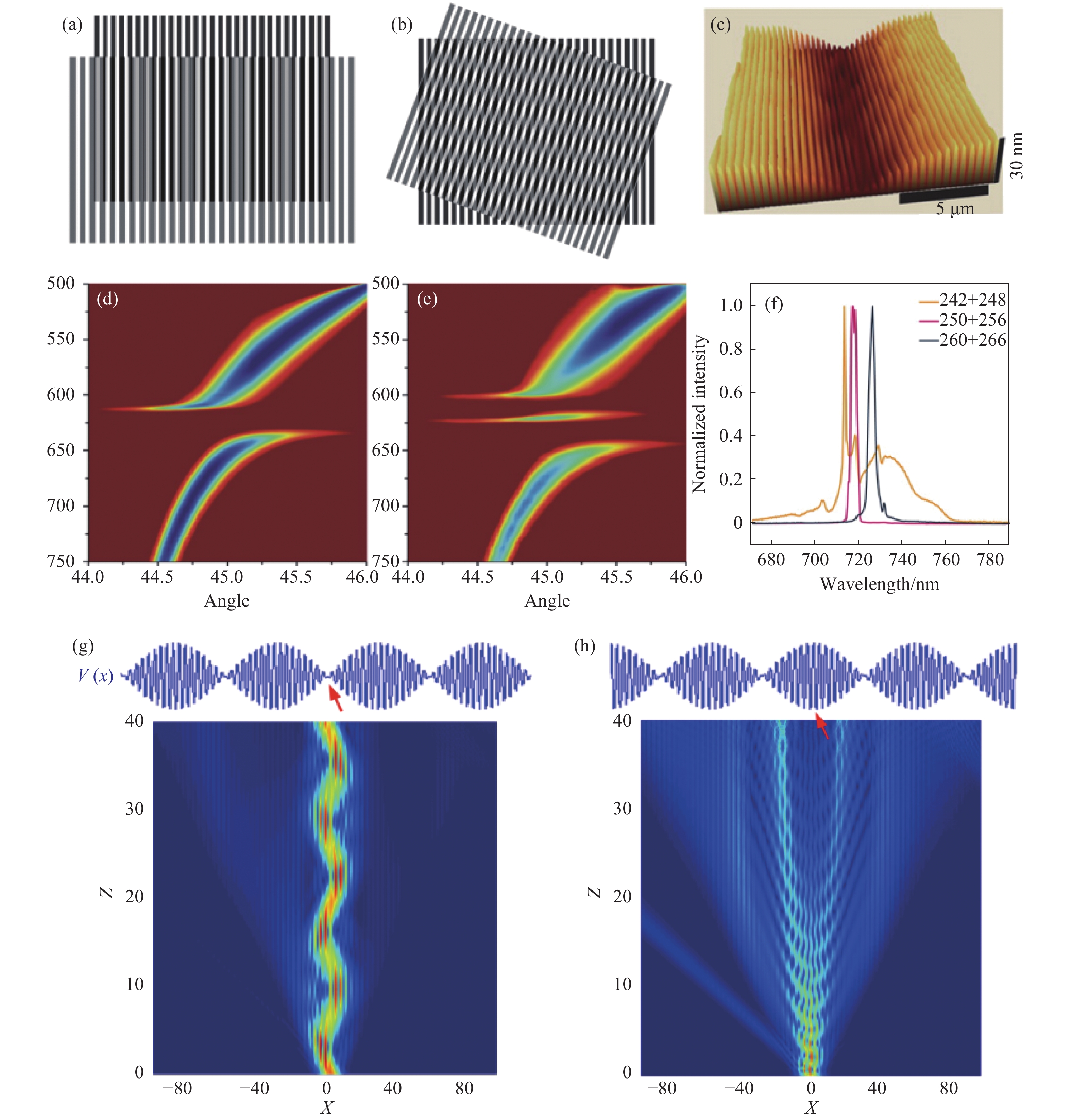
图 1  (a)由周期略有差异的两个一维光栅构成的莫尔光栅; (b)由两个周期相同的一维光栅，旋转一定角度后构成的二维莫尔光栅；(c)一个莫尔周期内的一维金属莫尔结构形貌图^[32]；（d，e）实验测得的金属光栅的SPP色散曲线和金属莫尔光栅的SPP色散曲线^[30]；(f)两个金属子光栅的周期（即d₁，d₂）发生改变时，相应的金属莫尔微腔辐射的激光发生频移^[32]；在一维莫尔晶格的节点(g)和最大包络处 (h)激发莫尔晶格，光在晶格中的传输图像^[34]。

Figure 1.  (a) Moiré gratings constituted from two 1D gratings with slightly detuned periods. (b) 2D moiré pattern constituted from two 1D gratings with a relative rotation angle. (c) Landscape of 1D metallic moiré structure shown within one moiré period ^[32]. (d,e) Experimentally measured dispersion curves of SPP on metallic 1D gratings and on moiré gratings ^[30]. (f) With the change of the period (i.e. d₁, d₂) of the two constituent metallic gratings, the lasing frequency of the corresponding metallic moiré cavity changes ^[32]. Light propagating dynamics when a 1D moiré lattice is optically excited at its node (g) or at its peak (h) of the structural envelop ^[34].

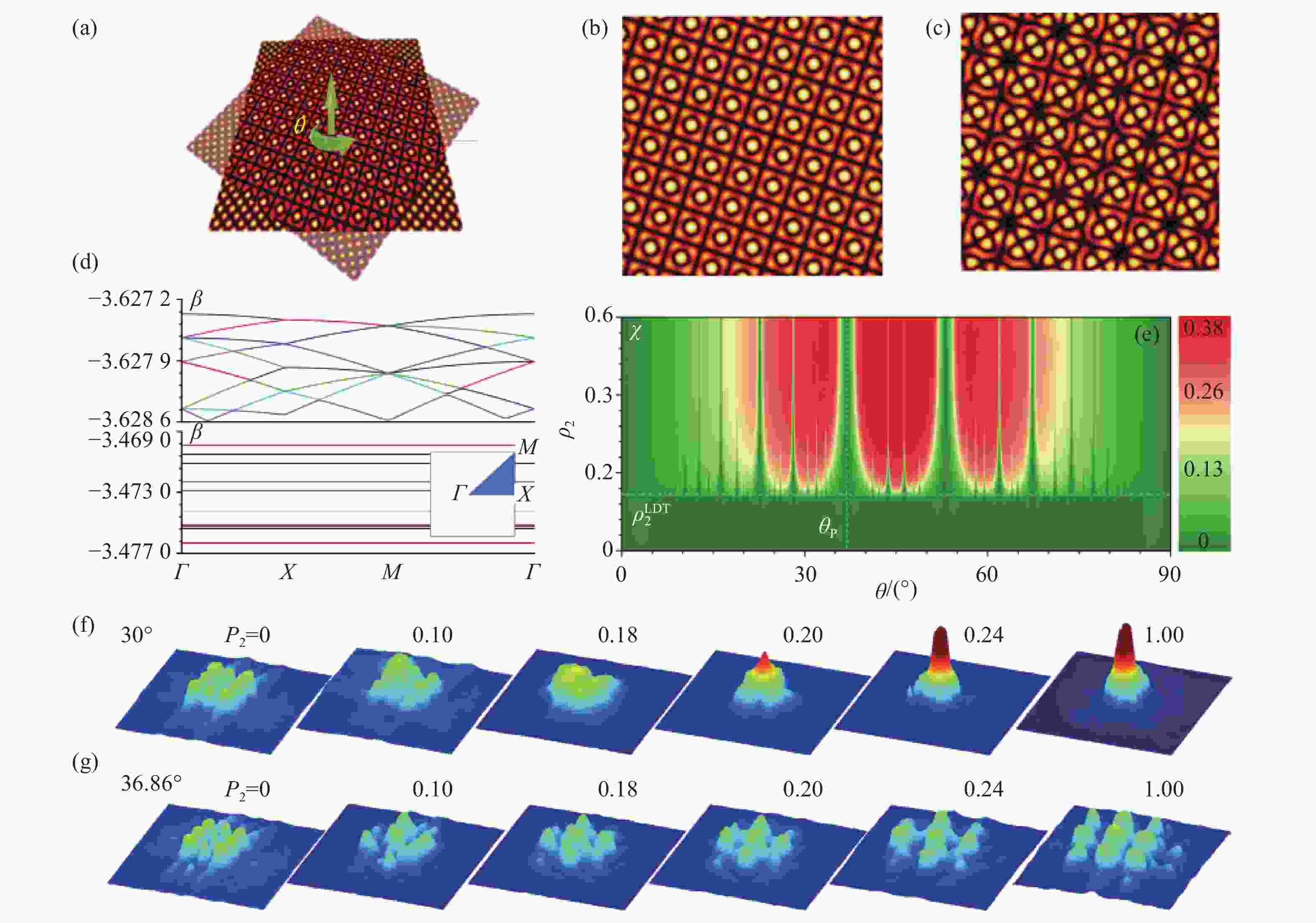
图 2  (a)由两个方形子晶格重叠，且转过相对角度θ后，构成的二维光子莫尔晶格；(b)周期莫尔晶格；(c)非周期莫尔晶格；(d)非周期莫尔晶格对应的准能带图，上图p₂<p₂^cr, 下图p₂>p₂^cr；(e) 本征模在二维平面（θ，p₂）的形式因子分布图，图中绿色代表模式处于非局域状态，红色代表模式处于局域状态；（f,g）用高斯光激发莫尔晶格，在不同p₂（固定p₁≡1）对应的晶格中传输2 cm后的光强输出图。上行图代表光束在非周期莫尔晶格中传输的情形，可以看到当p₂超过0.18（p₂^cr=0.15）时，光束开始局域；下行图代表光束在周期莫尔晶格中传输的情形，可以看到光束始终处于离散衍射的状态^[20]。

Figure 2.  (a) 2D photonic moiré lattices obtained by superpositioning two square lattices with a relative rotational angles θ; (b) A moiré lattice in a commensurate phase; (c) A moiré lattice in an incommensurate phase; (d) Quasi-bandstructure for an incommensurate moiré lattice: upper row for p₂<p₂^cr, lower row for p₂>p₂^cr；(e) Form factor of the eigen modes shown in the plane (θ, p₂), where the blue-colored domains imply mode delocalization, while the red-colored domains imply mode localization; (f, g) After propagating 2 cm in the moiré lattice of an initially narrow Gaussian beam, the lightintensity distribution at the output facet of the lattice with different p₂(with fixed p₁≡1). Upper row shows the results in incommensurate moiré lattices, and one can see that the light beam starts localizing when p₂ exceeds 0.18 (p₂^cr=0.15); Bottom row shows the results in commensurate moiré lattices, and one sees the delocalization of the light persists for all values of p₂^[20].

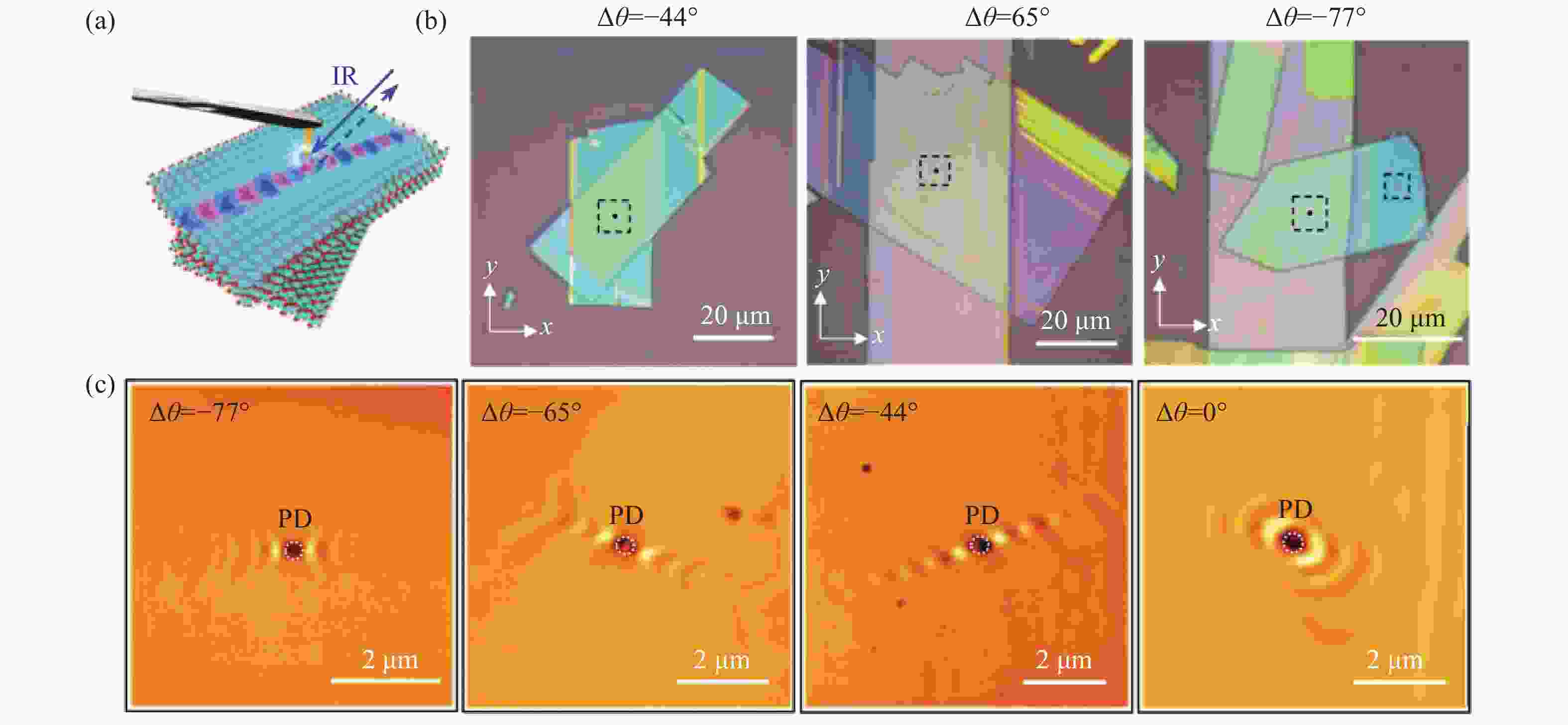
图 3  (a) 两层α-MoO₃堆叠构成的莫尔超晶格结构；(b)α-MoO₃莫尔超晶格结构在不同莫尔角下的光学照片；(c)声极化激元在不同莫尔角度下的波形分布图，显示出双曲-平带-椭圆三类拓扑上不同的色散带的转变^[55]。

Figure 3.  (a) Moiré superlattice constituted by twist-stacked α-MoO₃ bilayer; (b)Optical images of α-MoO₃ moiré superlattice as a function of rotation angle;(c) In-plane propagation of phonon polaritons at different twist angle of an α-MoO₃ bilayer, indicating a transition among three topologically different dispersion:hyperbolic, flat and elliptic ^[55].

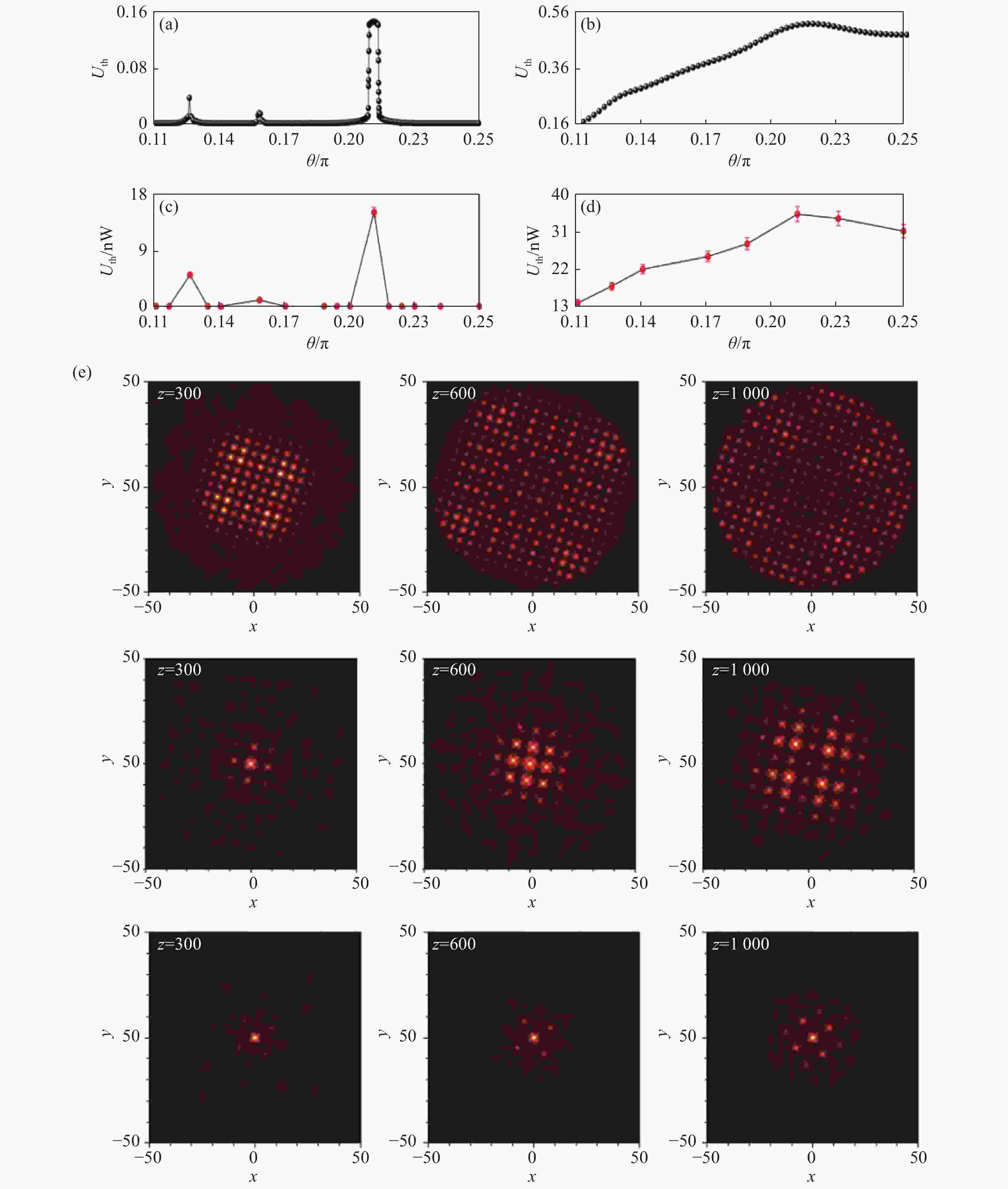
图 4  （a-d）激发莫尔光孤子的阈值功率随莫尔角的变化，（a,c）对应p₂>p₂^cr的情形，（b,d）对应p₂<p₂^cr的情形。（a，b）是模拟结果，（c，d）是实验结果^[21]。(e)给出了在莫尔角为三个不同勾股角时，莫尔晶格中光的衍射程度的比较。从上往下分别对应勾股角（3，4，5），（5，12，13），（8，5，17）。可以看到，随着勾股角的级次增加，光在莫尔晶格中的衍射效应不断降低，从而解释了图（a，c）中孤子阈值功率随着勾股角级次的增加不断降低的现象。

Figure 4.  (a-d) Dependencies of the threshold power on rotational angle for optical soliton formation in moiré lattices, (a, c) p₂>p₂^cr, (b, d) p₂<p₂^cr. Theoretically calculated results are shown in (a, c) and experimentally measured results are shown in(b, d) ^[21]. (e) Comparison of diffraction strength of light propagating in moiré lattices for three different Pythagorean angles, corresponding, from upper to bottom, to (3,4,5), (5,12,13), (8,5,17). Note the continuous decreasing of the light diffraction strength with the increasing of the order of Pythagorean triples, which explains the continuous decrease of the soliton threshold power with the Pythagorean angles seen in figure (a, c).