Optimization design and test of a high-precision measuring device of liquid refractive index based on the method of minimum deviation angle
-
摘要:
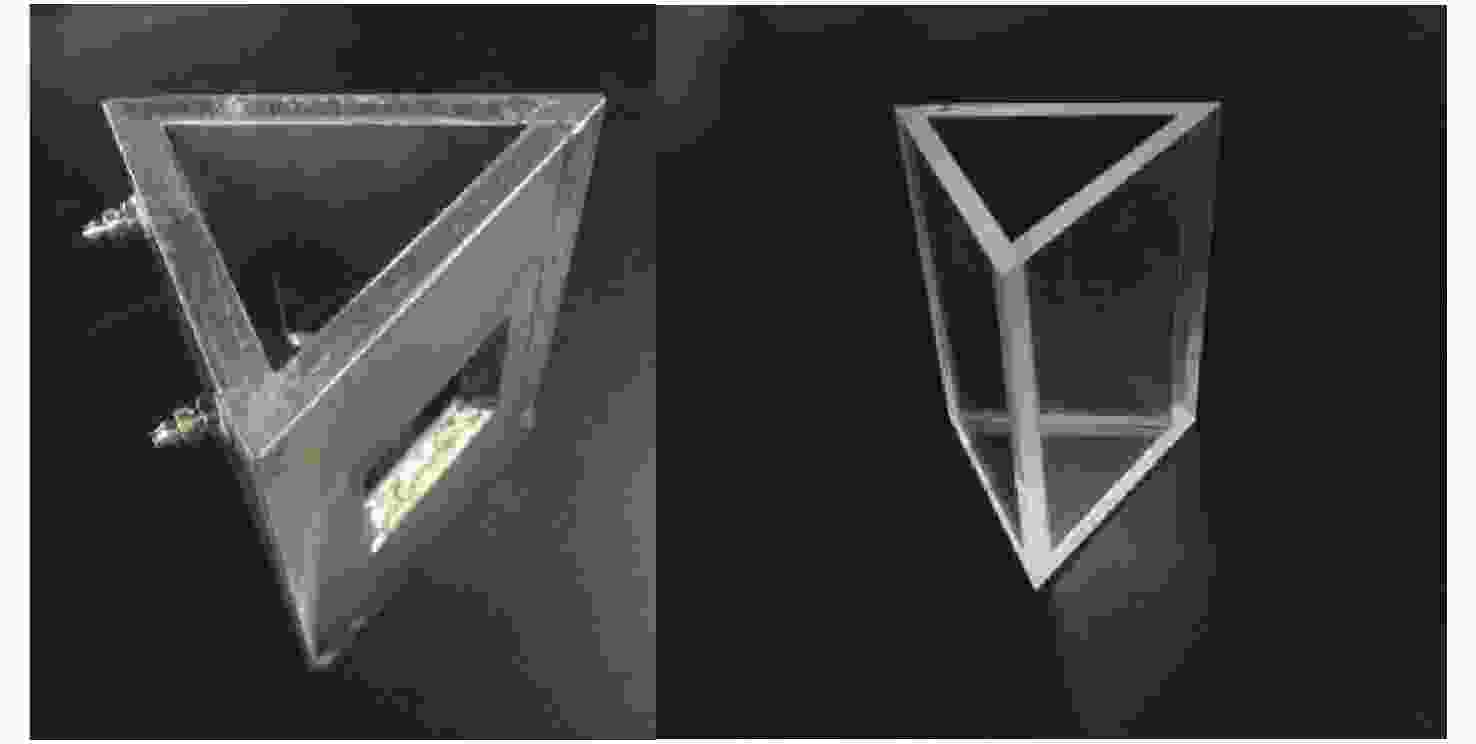
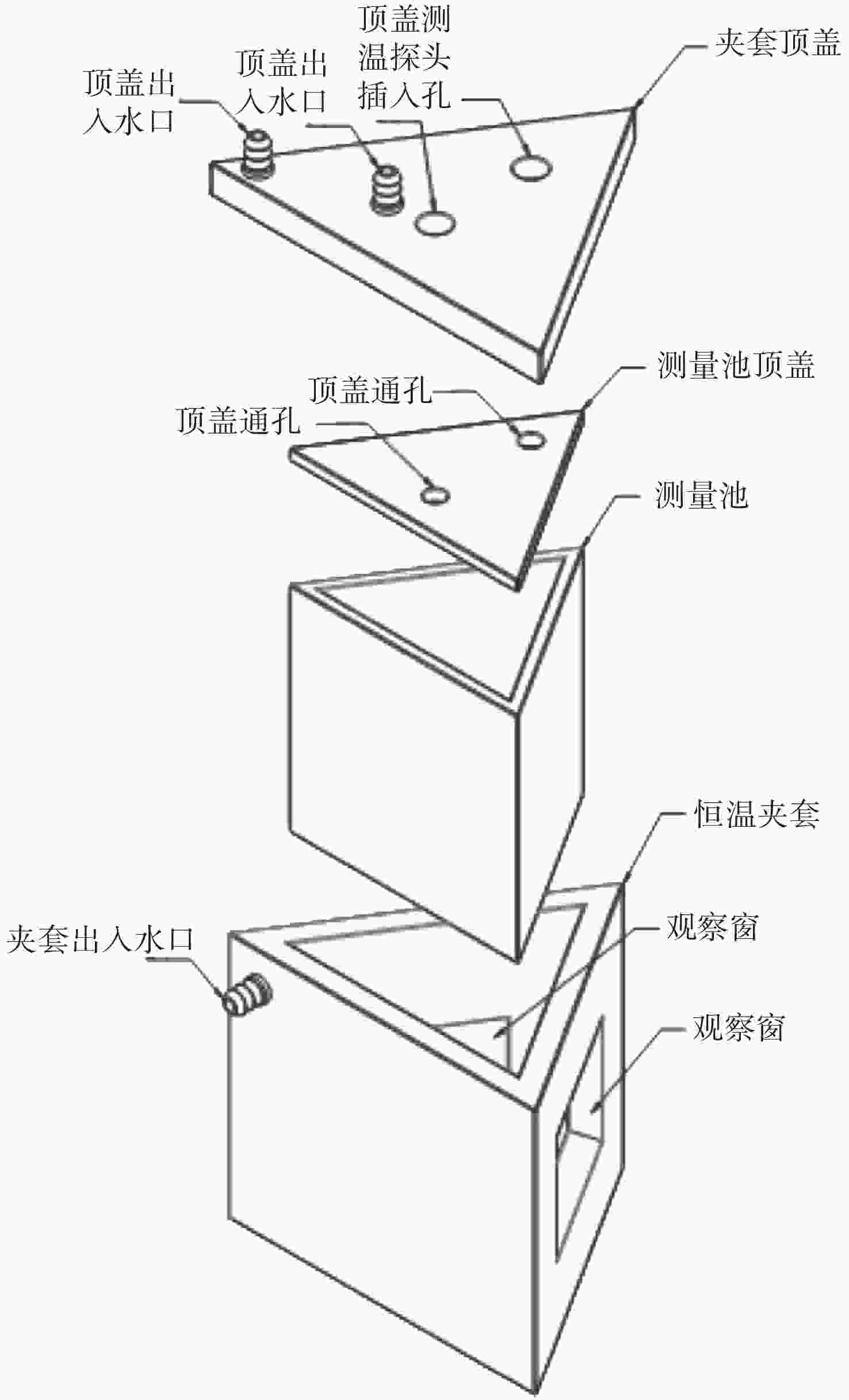
为了实现利用最小偏向角法对无定形流体的高精度折射率测量，设计了一种全新的恒温空心三棱镜装置，对该装置的光路和恒温组件进行精确设计，将其应用于测量液体折射率，对测量结果和不确定度进行定量分析。首先，通过对三棱镜光学平面的精确设计和加工，实现对测量光线的精准控制。其次，通过对恒温夹套内空心管路的迂回设计，使测量池内液体的温度波动和温场均匀性满足高精度折射率测量要求。最后，将该装置应用于液体折射率测量，定量分析了各影响因素的测量不确定度。实验结果表明：对于水、异辛烷、四氯乙烯3种液体，其折射率测量精度达到10−7，测量不确定度可低至10−5。实现了用最小偏向角法对液体折射率的高精度测量。
Abstract:For high-precision refractive index measurements of amorphous fluids, the minimum deviation angle method was used to design a novel thermostatic hollow trigonal prism device. The optical path and thermostatic compenents of the device are precisely designed. The device can be used not only to measure the refractive index of liquids, but also to quantify the measurement results and uncertainties. Firstly, the precise design and machining of the optical plane helps to precisely control the measurement light. Secondly, the tortuous hollow tube inside the thermostatic jacket is designed, which allows temperature fluctuations and uniformity of the liquid to be sufficient for high-precision refractive index measurements. Finally, the device is applied to measure a liquid’s refractive index, and the measurement uncertainty of each influence factor is quantitatively analyzed. The experimental results show that the refractive index measurement of three liquids, namely water, isooctane and tetrachloroethylene, could achieve an accuracy of 10−7 at 10−5 of uncertainty. Thus, the device provides a method for highly-precise measurements of the refractive index of liquids.
-
图 4 恒温夹套平面示意图。（a）无测量窗的侧面；（b）有测量窗的侧面；（c）顶面和底面
Figure 4. The planar graph of thermostatic inner layer. (a) Side without measuring window; (b) side with measuring window; (c) top and bottom
表 1 实验仪器
Table 1. Experimental instruments
名称 型号 厂商 高精度手动折射率
测量仪SpectroMaster® TRIOPTICS 高精度台式液体折射仪 Abbemat 550 奥地利安东帕公司 手持式参考测温仪 1524 美国福禄克公司 空心三棱镜 / 苏州诺威特测控科技
有限公司数字气压表 370 美国Setra公司 温湿度计 608-H1 德图仪表（深圳）有限公司 恒温水浴 RTS-0AT 湖州唯立仪表厂 
下载: 导出CSV
表 2 实验试剂
Table 2. Experimental reagent
名称 级别或标准号 生产厂家 纯水 电阻值大于18 MΩ 自制 异辛烷 纯度99.8% 阿拉丁 四氯乙烯 纯度≥99% 阿拉丁
下载: 导出CSV
表 3 液体折射率的实验数据与统计学分析
Table 3. Experimental data and statistical analysis of liquid refractive index
待测液体 目标温度（ °C） 1 2 3 4 5 6 平均值
$ \overline {{n_x}} $标准偏差S 水 15.00 1.3333632 1.3333648 1.3333695 1.3333654 1.3333665 1.3333647 1.3333657 2.16×10−6 20.00 1.3329905 1.3329922 1.3329929 1.3329924 1.3329916 1.3329918 1.3329919 8.25×10−7 25.00 1.3325078 1.3325084 1.3325059 1.3325063 1.3325068 1.3325080 1.3325072 1.01×10−6 异辛烷 15.00 1.3939165 1.3939148 1.3939190 1.3939154 1.3939136 1.3939173 1.3939161 1.92×10−6 20.00 1.3914694 1.3914681 1.3914691 1.3914691 1.3914695 1.3914681 1.3914689 6.27×10−7 25.00 1.3890532 1.3890495 1.3890541 1.3890508 1.3890477 1.3890510 1.3890511 2.35×10−6 四氯乙烯 15.00 1.5084995 1.5085050 1.5085023 1.5084986 1.5084993 1.5085052 1.5085017 2.96×10−6 20.00 1.5057895 1.5057887 1.5057908 1.5057941 1.5057903 1.5057909 1.5057907 1.86×10−6 25.00 1.5031255 1.5031256 1.5031264 1.5031251 1.5031268 1.5031262 1.5031259 6.38×10−7
下载: 导出CSV
表 4 两种不同原理的测量结果
Table 4. Comparative results of two different principles
待测液体 最小偏向角法
测量平均值全反射角法
测量平均值折射率
测量偏差水 1.3329919 1.332993 −1.1×10−6 异辛烷 1.3914689 1.391467 1.9×10−6 四氯乙烯 1.5057907 1.505790 7.0×10−7
下载: 导出CSV
表 5 环境温度下的液体折射率
Table 5. Refractive indices of liquid at ambient temperature
测温( °C) 18.97 19.75 19.92 19.99 20.02 水 1.3330691 1.3330121 1.3329953 1.3329932 1.3329896 (20±1) °C时，水折射率随温度变化系数：−7.6×10−5/°C 测温(°C) 20.04 20.14 20.32 20.40 20.77 异辛烷 1.3914895 1.3913984 1.3913075 1.3912616 1.3910314 (20±1) °C时，异辛烷折射率随温度变化系数：−6.1×10−4/ °C 测温(°C) 19.20 19.31 19.90 19.98 20.08 四氯乙烯 1.5062404 1.5062193 1.5058509 1.5058002 1.5057544 (20±1) °C时，四氯乙烯折射率随温度变化系数：−5.8×10−4/°C
下载: 导出CSV
表 6 空气折射率引入的标准不确定度分量
Table 6. Standard uncertainty introduced by air refractive index
待测液体 $ u({n_0}) $ $ {n_x} $ u1 水 3.46×10−6 1.3329932 4.62×10−6 异辛烷 3.46×10−6 1.3914727 4.82×10−6 四氯乙烯 3.46×10−6 1.5058002 5.22×10−6
下载: 导出CSV
表 7 测温误差引入的标准不确定度
Table 7. Standard uncertainty introduced by temperature measurement error
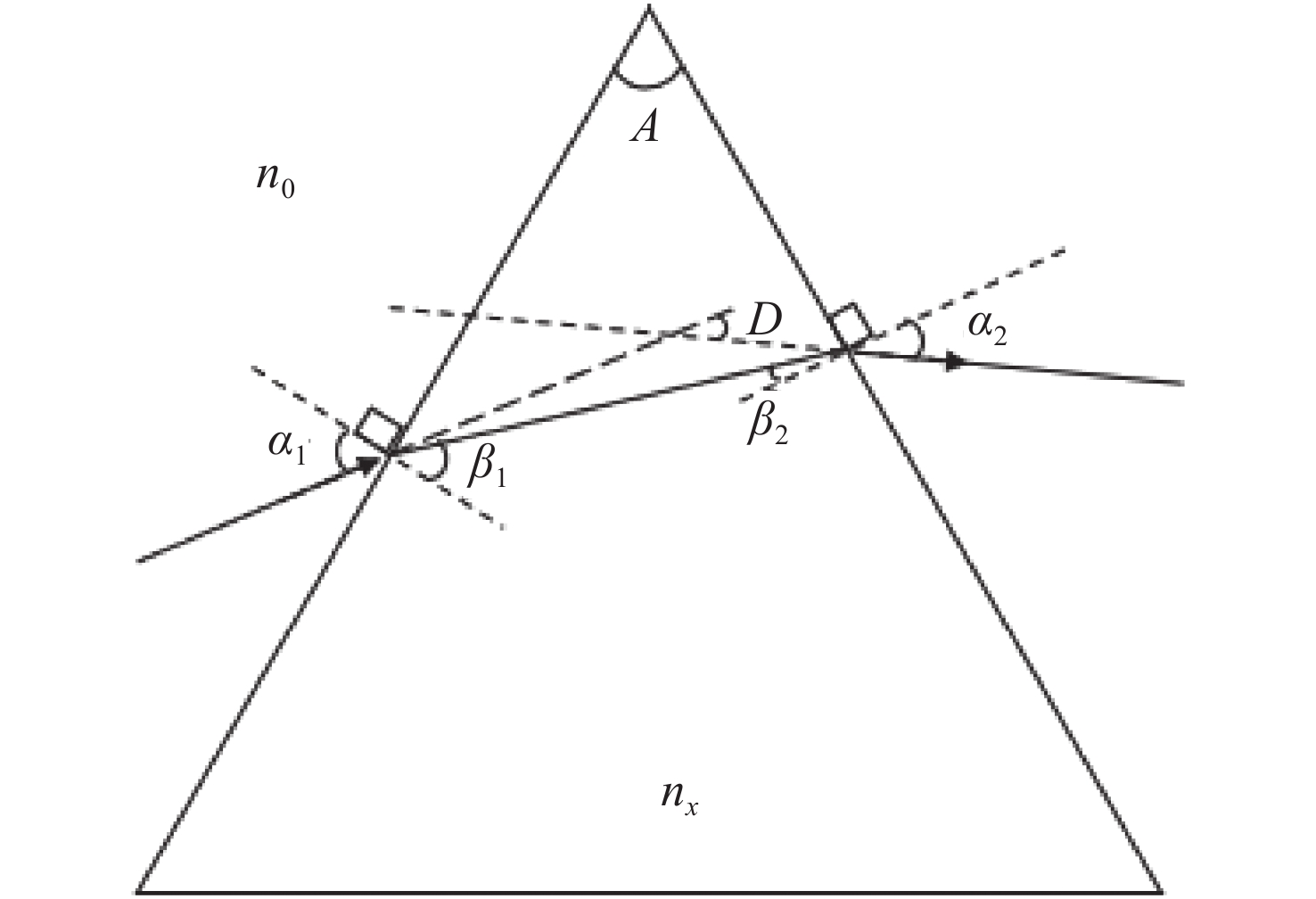
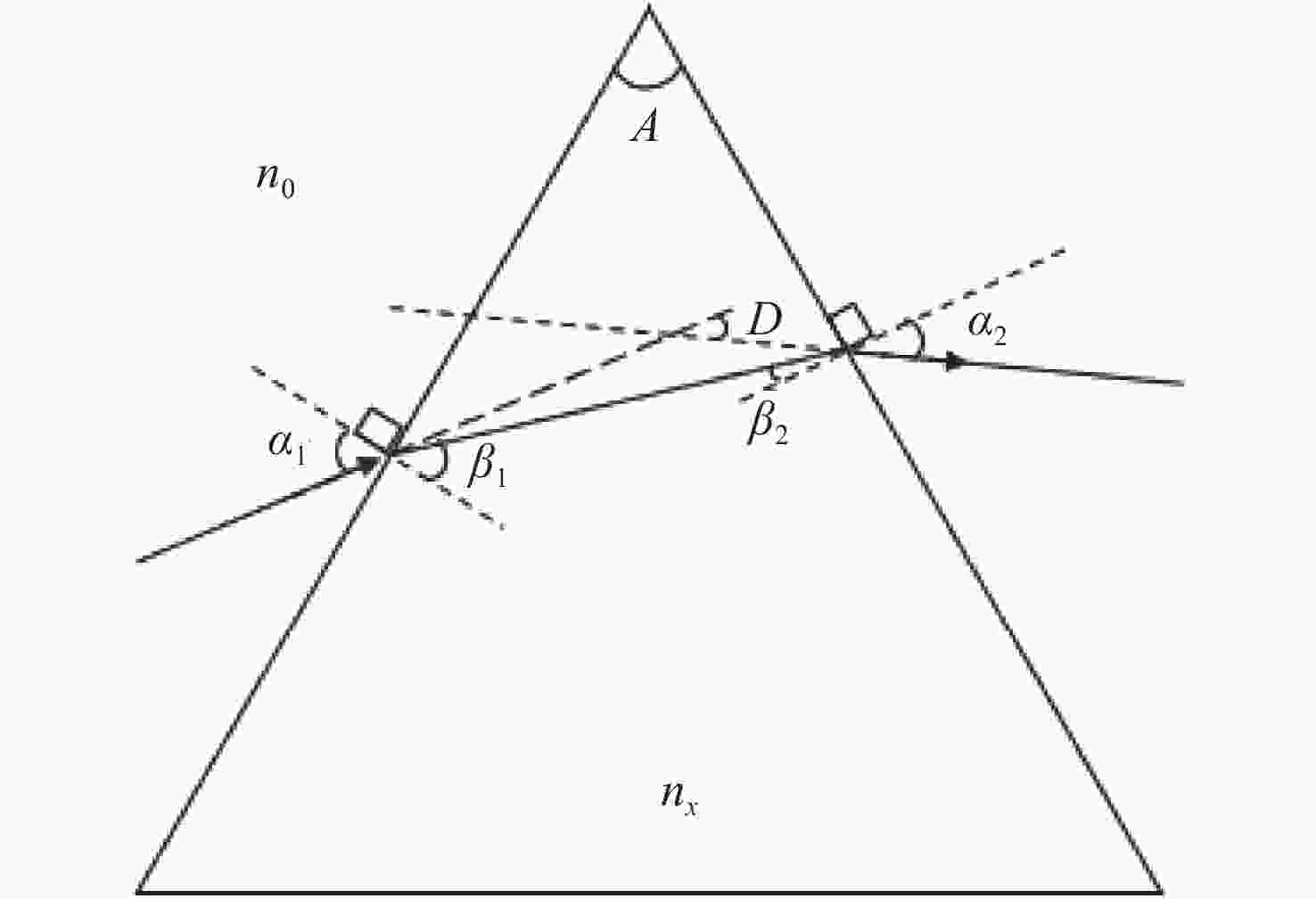
待测液体 a b $ {c_t} $ $ {u_2} $ 水 −8.11×10−5 −3.63×10−6 −8.11×10−5 −1.40×10−6 异辛烷 −4.84×10−4 −4.72×10−6 −4.84×10−4 −8.39×10−6 四氯乙烯 −5.48×10−4 1.25×10−4 −5.48×10−4 −9.49×10−6
下载: 导出CSV
表 8 顶角及最小偏向角实验数据
Table 8. Experimental data of vertex angle and minimum deviation angle
待测液体 顶角A 最小偏向角D $ {n_x} $ 水 54.99965° 20.97748° 1.3329932 异辛烷 54.99972° 24.95978° 1.3914727 四氯乙烯 54.99970° 33.10221° 1.5058002
下载: 导出CSV
表 9 角度测量误差引入的标准不确定度分量
Table 9. Standard uncertainty introduced by angle measurement error
待测液体 $ {u_{{\rm{rad}}}} $ $\dfrac{ {\partial {n_x} } }{ {\partial A} }$ $\dfrac{ {\partial {n_x} } }{ {\partial D} }$ $ {u_3} $ 水 1.41×10−6 4.27 1.71 6.46×10−6 异辛烷 1.41×10−6 4.33 1.66 6.52×10−6 四氯乙烯 1.41×10−6 4.45 1.56 6.63×10−6
下载: 导出CSV
表 10 不确定度分量及合成不确定度
Table 10. Uncertainty component and combined uncertainty
待测液体 $ u_s^{} $ $ {u_1} $ $ {u_2} $ $ {u_3} $ $ u({n_x}) $ 水 3.37×10−7 4.62×10−6 −1.40×10−6 6.46×10−6 8.1×10−6 异辛烷 2.56×10−7 4.82×10−6 −8.39×10−6 6.52×10−6 1.2×10−5 四氯乙烯 7.59×10−7 5.22×10−6 −9.48×10−6 6.63×10−6 1.3×10−5
下载: 导出CSV
-
[1] 唐云辉. 高折射率光学树脂的分子设计、合成及应用研究[D]. 北京: 北京化工大学, 2019.TANG Y H. Molecular design, synthesis and application of high refractive index optical resin[D]. Beijing: Beijing University of Chemical Technology, 2019. (in Chinese) [2] 任明阳, 王立忠, 付白强, 等. 高温数字图像相关法变形测量中玻璃介质误差校正[J]. 中国光学,2022,15(2):327-338. doi: 10.37188/CO.2021-0144REN M Y, WANG L ZH, FU B Q, et al. Error correction of glass mediums in high-temperature digital image correlation deformation measurement[J]. Chinese Optics, 2022, 15(2): 327-338. (in Chinese) doi: 10.37188/CO.2021-0144 [3] 张佳伦, 郑玉权, 蔺超, 等. 消像散的自由曲面棱镜光谱仪光学系统设计[J]. 中国光学,2020,13(4):842-851. doi: 10.37188/CO.2019-0049ZHANG J L, ZHENG Y Q, LIN CH, et al. Design of a freeform curved prism imaging spectrometer based on an anastigmatism[J]. Chinese Optics, 2020, 13(4): 842-851. (in Chinese) doi: 10.37188/CO.2019-0049 [4] 张国强, 赵鸣, 张丽娟, 等. 激光粒度测试结果影响因素分析[J]. 中国测试,2017,43(3):24-29. doi: 10.11857/j.issn.1674-5124.2017.03.006ZHANG G Q, ZHAO M, ZHANG L J, et al. Analysis of influencing factors on laser particle size measurement[J]. China Measurement &Test, 2017, 43(3): 24-29. (in Chinese) doi: 10.11857/j.issn.1674-5124.2017.03.006 [5] 高萍萍, 陆敏, 王治乐, 等. 表面纳米粒子缺陷的偏振散射特性区分[J]. 中国光学,2020,13(5):975-987. doi: 10.37188/CO.2020-0083GAO P P, LU M, WANG ZH L, et al. Differentiation of polarization scattering characteristics of surface nanoparticle defects[J]. Chinese Optics, 2020, 13(5): 975-987. (in Chinese) doi: 10.37188/CO.2020-0083 [6] 韦秋叶, 范晓辉. 液体折射率的测量方法研究进展[J]. 计量科学与技术,2022,66(1):14-18.WEI Q Y, FAN X H. Review of methods for measuring liquid refractive index[J]. Metrology Science and Technology, 2022, 66(1): 14-18. (in Chinese) [7] HIRAI A, HORI Y, MINOSHIMA K, et al. A bilateral comparison of optical glass refractive index between NMIJ and INRiM for the validation of the measuring systems[J]. Metrologia, 2012, 49(3): 283-288. doi: 10.1088/0026-1394/49/3/283 [8] WU G H, ARAI K, TAKAHASHI M, et al. High-accuracy correction of air refractive index by using two-color heterodyne interferometry of optical frequency combs[J]. Measurement Science and Technology, 2013, 24(1): 015203. doi: 10.1088/0957-0233/24/1/015203 [9] MENG ZH P, ZHAI X Y, WEI J G, et al. Absolute measurement of the refractive index of water by a Mode-Locked laser at 518 nm[J]. Sensors, 2018, 18(4): 1143. doi: 10.3390/s18041143 [10] 陈余行, 马振斌. 最小偏向角法测量NaCl溶液浓度与折射率[J]. 实验科学与技术,2010,8(6):16-17,112. doi: 10.3969/j.issn.1672-4550.2010.06.007CHEN Y X, MA ZH B. Research on the refractive index of NaCl solution by minimum angle of deviation[J]. Experiment Science and Technology, 2010, 8(6): 16-17,112. (in Chinese) doi: 10.3969/j.issn.1672-4550.2010.06.007 [11] 杨春章, 薄晓红. 最小偏向角法测量光学玻璃的折射率[J]. 计量技术,1991(2):12-13,45.YANG CH ZH, BO X H. Measurement of refractive index of optical glass by minimum deflection angle method[J]. Metrology Technology, 1991(2): 12-13,45. (in Chinese) [12] 孙一书, 陈怡, 韩冰, 等. 应用最小偏向角法的液体折射率精密测试[J]. 中国光学,2019,12(4):826-832. doi: 10.3788/co.20191204.0826SUN Y SH, CHEN Y, HAN B, et al. Precision test technology of liquid refractive index using the method of minimum deviation angle[J]. Chinese Optics, 2019, 12(4): 826-832. (in Chinese) doi: 10.3788/co.20191204.0826 [13] MILLIARD R C, SEAVER G. An index of refraction algorithm for seawater over temperature, pressure, salinity, density, and wavelength[J]. Deep Sea Research Part A. Oceanographic Research Papers, 1990, 37(12): 1909-1926. doi: 10.1016/0198-0149(90)90086-B [14] Physikalisch-Technische Bundesanstalt. Calibration certificate: 1659 PTB 14[S]. 2014. -
下载:
计量
- 文章访问数: 987
- HTML全文浏览量: 297
- PDF下载量: 125
- 被引次数: 0
