Averaged intensity and spectral shift of partially coherent chirped optical coherence vortex lattices in biological tissue turbulence
doi: 10.37188/CO.EN.2021-0010
-
摘要: 本文研究了部分相干啁啾光学相干涡旋晶格在生物组织湍流中的平均光强和光谱位移，详细探讨了单色光场中的光学晶格结构和多色光场中的光谱快速跃迁特性。研究表明：在生物组织湍流中，光束从具有涡旋核的环形结构演变为具有暗区的周期阵列结构，最后呈类高斯图样。尽管晶格常数能调制光束结构，但它不影响光束在生物组织湍流中的光谱跃迁行为。光束在生物组织中的传输距离越小越有利于光谱快速跃迁，并且随着啁啾参数增大和脉冲持续时间的减小会导致发生光谱快速跃迁的横坐标减小。光谱跃迁和光谱位移会受到生物组织湍流的长距离累积效应的压制，并且湍流越强，其光谱位移越小。本文所得结果对生物组织中的图像识别、医疗器械和无损光学诊疗技术具有潜在应用。Abstract: In this paper, an averaged intensity and spectral shift of Partially Coherent Chirped Optical Coherence Vortex Lattices (PCCOCVLs) in biological tissue turbulence are investigated with emphasis on optical lattice structures in monochromatic optical fields and spectral rapid transitions in polychromatic optical fields. It is found that the beam profile evolves from annular structures with a vortex core into a periodic array of lobes with a dark zone, and finally presents a Gaussian-like pattern in biological tissue. Although lattice parameter modulates beam profile, it cannot affect the spectral behavior in biological tissue turbulence. The analysis of spectral shift also shows that a smaller distance is beneficial to spectral rapid transition where the transverse coordinate decreases with an increase in chirp parameter and a decrease in pulse duration. The accumulated turbulences over a longer distance can suppress not only spectral transition but also spectral shift. The reduction of spectral shift is accompanied by stronger biological tissue turbulence. The results have possible application in image recognition, medical devices and noninvasive optical diagnoses in biological tissue.
-
Key words:
- optical coherence lattices /
- biological tissue turbulence /
- spectral shift /
- vortex
-
Figure 1. Intensity evolution of PCCOCVL beams with monochromatic light in biological tissue for different lattices parameter a
Figure 2. For canonical and noncanonical lattices parameters, the intensity profile of PCCOCVL beams with monochromatic light in biological tissue for different topological charge m
Figure 3. Relative spectral shift of the PCCOCVLs versus the transverse coordinate x for different lattices parameter a
Figure 4. Relative spectral shift of the PCCOCVLs beam versus the transverse coordinate x for different C and T
Figure 5. Relative spectral shift of the PCCOCVL beam versus transverse coordinate x for different biological tissue turbulences
-
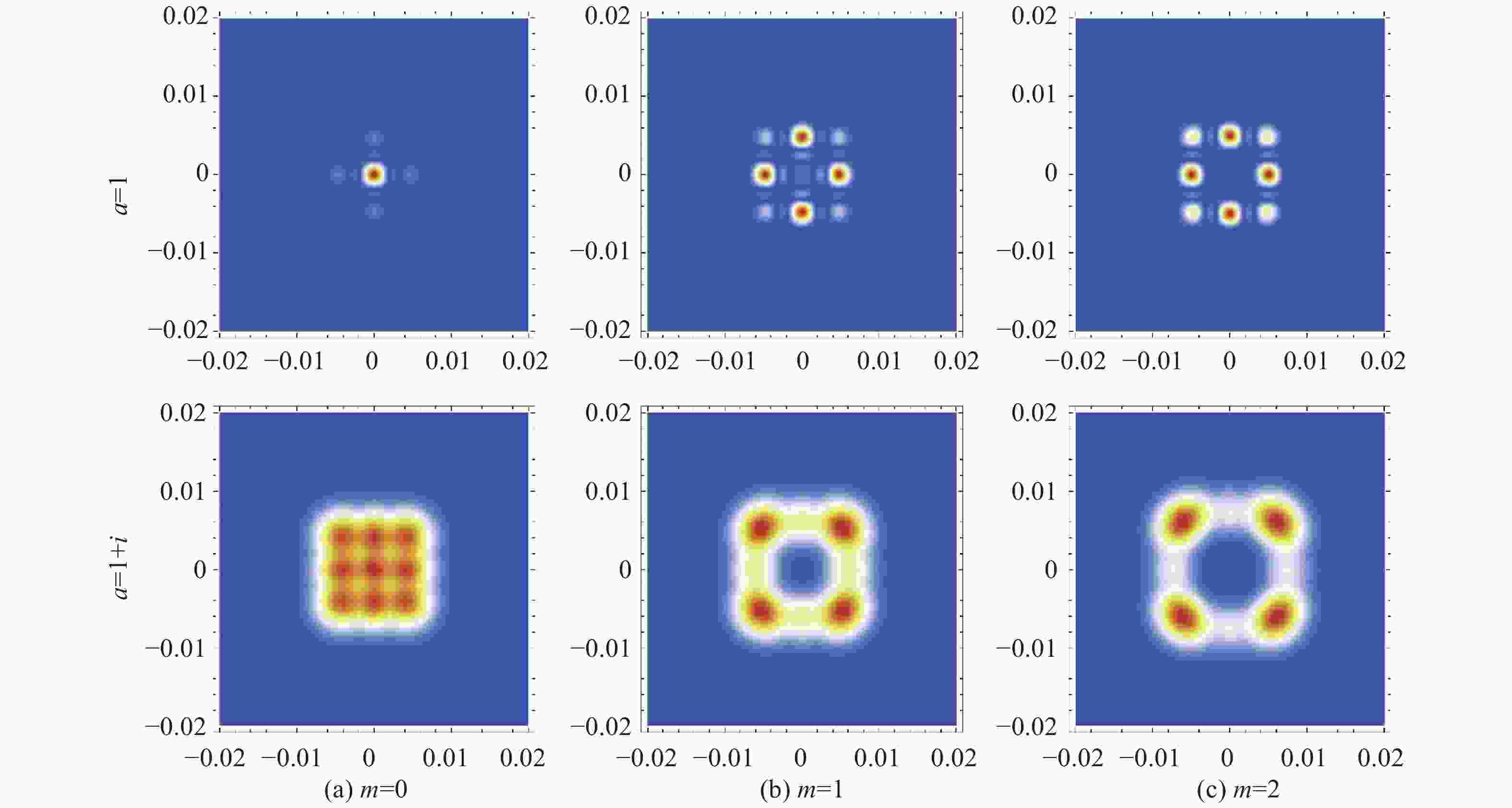
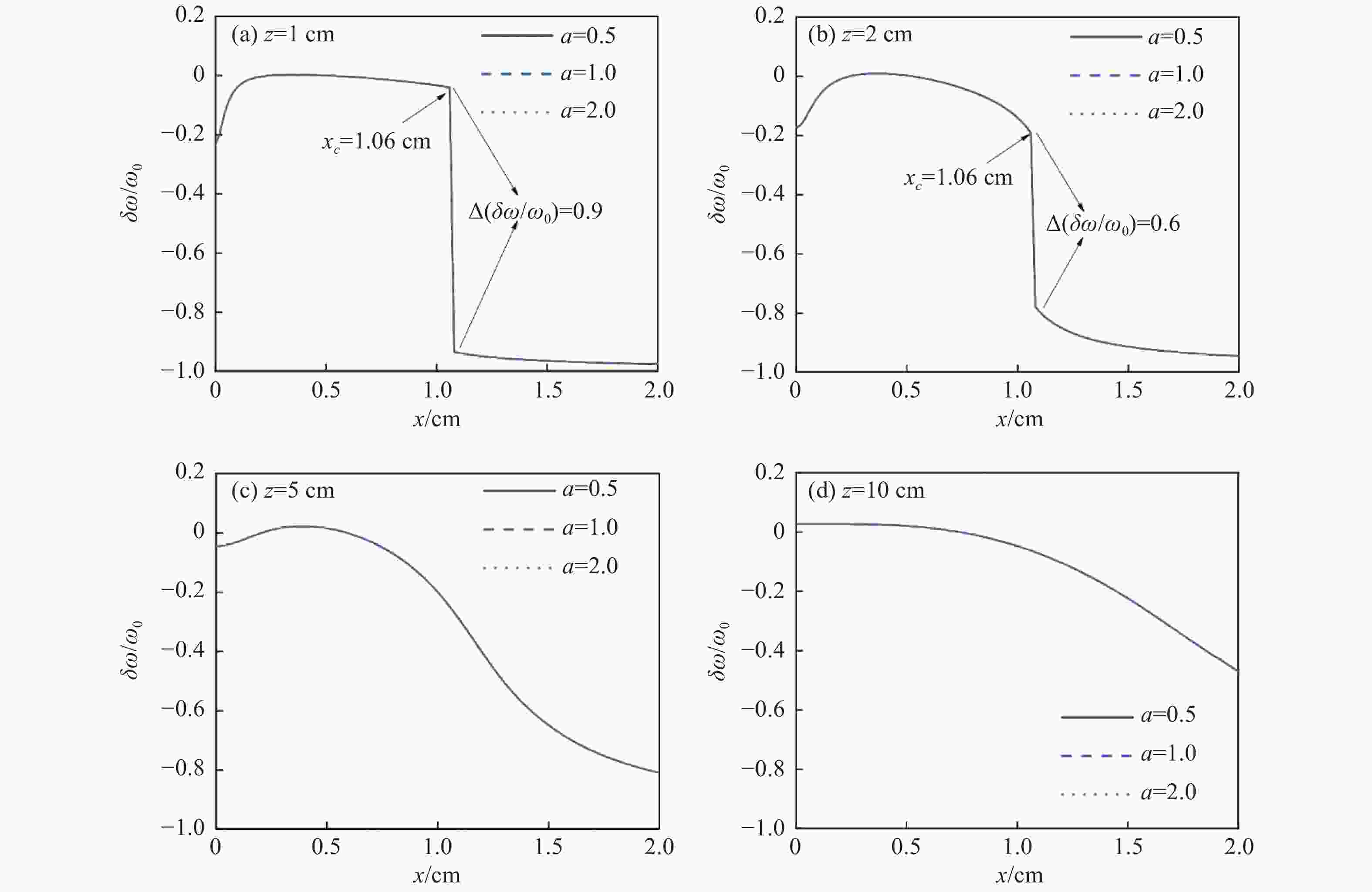
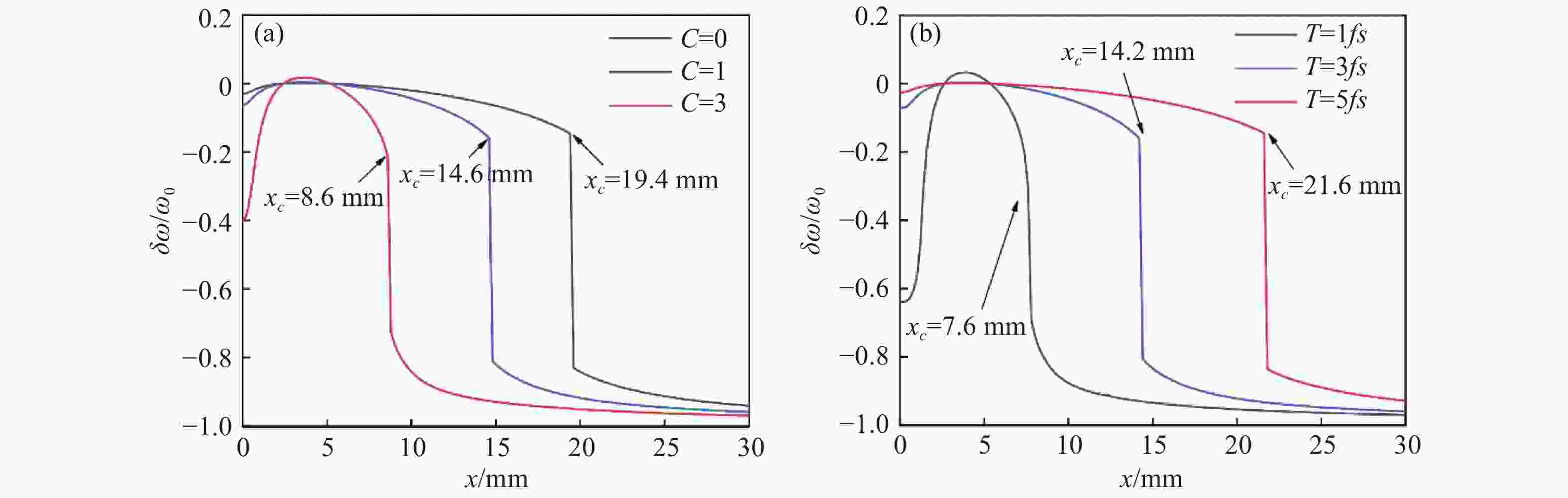
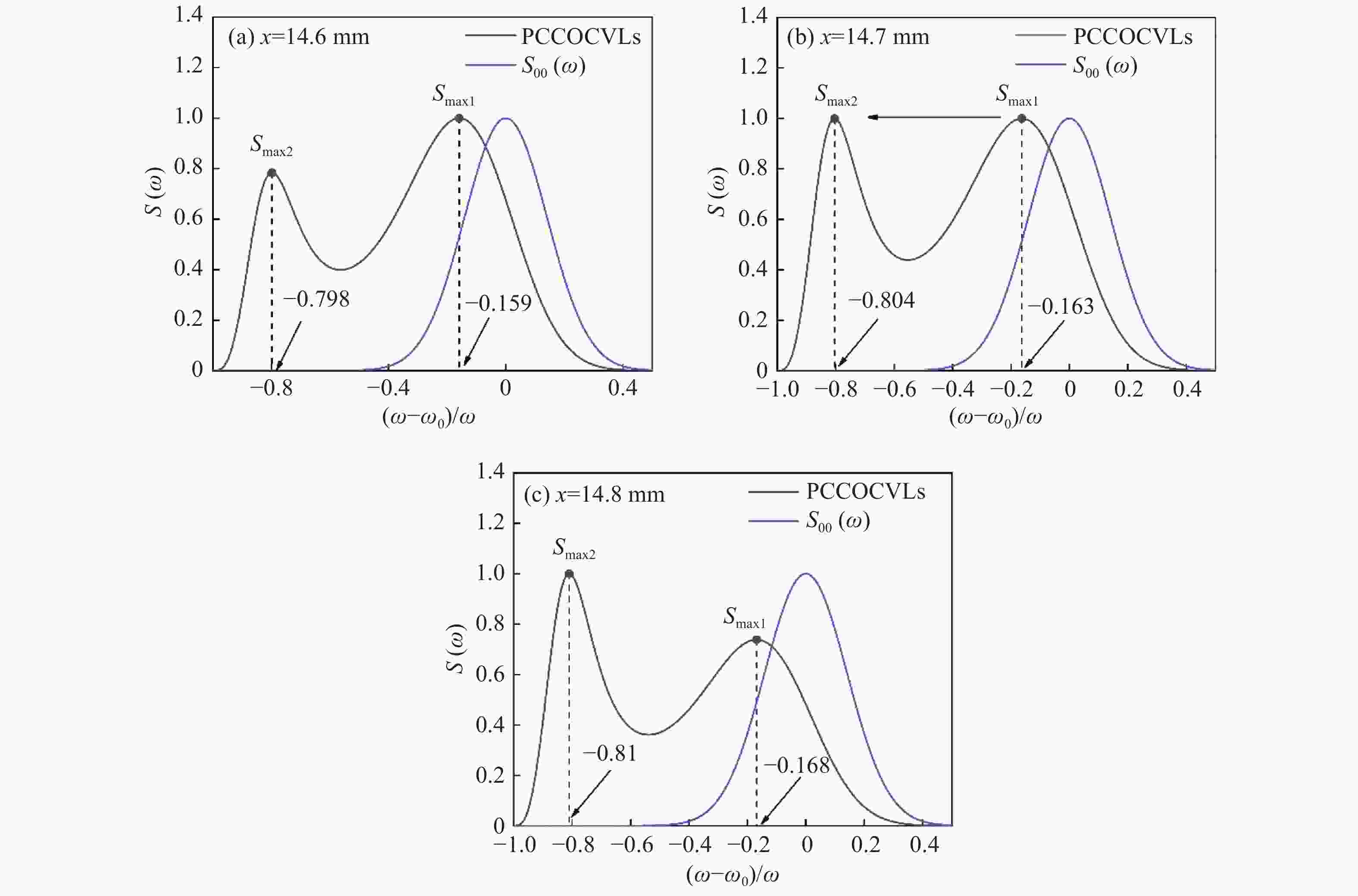
[1] SCHMITT J M, KUMAR G. Turbulent nature of refractive-index variations in biological tissue[J]. Optics Letters, 1996, 21(16): 1310-1312. doi: 10.1364/OL.21.001310 [2] GAO W R, KOROTKOVA O. Changes in the state of polarization of a random electromagnetic beam propagating through tissue[J]. Optics Communications, 2007, 270(2): 474-478. doi: 10.1016/j.optcom.2006.09.061 [3] LIU X Y, ZHAO D M. The statistical properties of anisotropic electromagnetic beams passing through the biological tissues[J]. Optics Communications, 2012, 285(21-22): 4152-4156. doi: 10.1016/j.optcom.2012.06.033 [4] ZHANG H H, CUI ZH W, HAN Y P, et al. Average intensity and beam quality of Hermite-Gaussian correlated Schell-Model beams propagating in turbulent biological tissue[J]. Frontiers in Physics, 2021, 9: 650537. doi: 10.3389/fphy.2021.650537 [5] MA L Y, PONOMARENKO S A. Optical coherence gratings and lattices[J]. Optics Letters, 2014, 39(23): 6656-6659. doi: 10.1364/OL.39.006656 [6] MA L Y, PONOMARENKO S A. Free-space propagation of optical coherence lattices and periodicity reciprocity[J]. Optics Express, 2015, 23(2): 1848-1856. doi: 10.1364/OE.23.001848 [7] CHEN Y H, PONOMARENKO S A, CAI Y J. Experimental generation of optical coherence lattices[J]. Applied Physics Letters, 2016, 109(6): 061107. doi: 10.1063/1.4960966 [8] LIU X L, YU J Y, CAI Y J, et al. Propagation of optical coherence lattices in the turbulent atmosphere[J]. Optics Letters, 2016, 41(18): 4182-4185. doi: 10.1364/OL.41.004182 [9] LUO B, WU G H, YIN L F, et al. Propagation of optical coherence lattices in oceanic turbulence[J]. Optics Communications, 2018, 425: 80-84. doi: 10.1016/j.optcom.2018.04.076 [10] HUANG Y, YUAN Y SH, LIU X L, et al. Propagation of optical coherence vortex lattices in turbulent atmosphere[J]. Applied Sciences, 2018, 8(12): 2476. doi: 10.3390/app8122476 [11] YE F, XIE J T, HONG SH H, et al. Propagation properties of a controllable rotating elliptical Gaussian optical coherence lattice in oceanic turbulence[J]. Results in Physics, 2019, 13: 102249. doi: 10.1016/j.rinp.2019.102249 [12] HUANG X W, DENG ZH X, SHI X H, et al. Average intensity and beam quality of optical coherence lattices in oceanic turbulence with anisotropy[J]. Optics Express, 2018, 26(4): 4786-4797. doi: 10.1364/OE.26.004786 [13] GUO M W, ZHAO D M. Interfering optical coherence lattices by use of a wavefront-folding interferometer[J]. Optics Express, 2017, 25(13): 14351-14358. doi: 10.1364/OE.25.014351 [14] LIANG CH H, MI CH K, WANG F, et al. Vector optical coherence lattices generating controllable far-field beam profiles[J]. Optics Express, 2017, 25(9): 9872-9885. doi: 10.1364/OE.25.009872 [15] JI X L, ZHANG E T, LÜ B D. Spectral properties of chirped Gaussian pulsed beams propagating through the turbulent atmosphere[J]. Journal of Modern Optics, 2007, 54(4): 541-553. doi: 10.1080/09500340600964080 [16] LIU D J, WANG G Q, WANG Y CH. Average intensity and coherence properties of a partially coherent Lorentz-Gauss beam propagating through oceanic turbulence[J]. Optics &Laser Technology, 2018, 98: 309-317. [17] DUAN M L, WU Y G, ZHANG Y M, et al. Coherence properties of a random electromagnetic vortex beam propagating in biological tissues[J]. Journal of Modern Optics, 2019, 66(1): 59-66. doi: 10.1080/09500340.2018.1511863 [18] ARPALI S A, ARPALI ç, BAYKAL Y. Bit error rate of a Gaussian beam propagating through biological tissue[J]. Journal of Modern Optics, 2020, 67(4): 340-345. doi: 10.1080/09500340.2020.1719226 [19] DUAN M L, TIAN Y N, ZHANG Y M, et al. Influence of biological tissue and spatial correlation on spectral changes of Gaussian-Schell model vortex beam[J]. Optics and Lasers in Engineering, 2020, 134: 106224. doi: 10.1016/j.optlaseng.2020.106224 -
下载:
图(6)
计量
- 文章访问数: 742
- HTML全文浏览量: 458
- PDF下载量: 87
- 被引次数: 0
