Measurement and calibration of the intensity transform function of the optical 3D profilometry system
-
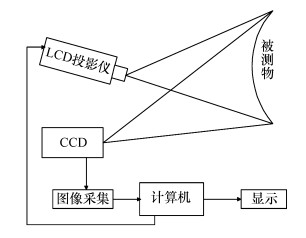
摘要: 由于数字光栅投影仪的光强传递函数对于正弦投影条纹的质量以及相位测量精度起着至关重要的作用,本文提出了一种校正光学三维扫描仪光强传递函数的新方法。首先,分析了由于投影仪非线性响应引起的光栅谐波的相位测量误差;然后,通过投影一组不同灰度级的图像,并利用光功率计测出数字投影仪投出图像的亮度。接着,通过分析得到数字投影仪的非线性响应特性曲线,再经过数据处理,即可获得投影仪的光强传递函数;最后,对光强传递函数进行反函数逆变换,得到一个校正后的非正弦光栅,利用投影仪对该光栅的投影即可在被测物体表面上获得一个正弦光栅。数字投影仪对标准平板的测量结果表明,校正前平均误差为0.71 mm,校正后为0.55 mm;对于标准量块的测量,校正前的平均误差为0.62 mm,校正后为0.15 mm。上述结果表明,本文提出的方法可以减小由于系统非线性响应引起的测量误差并提高测量精度。Abstract: The light intensity transform function(ITF) of the digital grating projector plays a crucial role in the quality of sinusoidal projection fringes and the accuracy of phase measurement. Based on this, a new method of correcting the ITF of digital grating projector in optical 3D profilometry system is proposed in this paper. Firstly, the phase measurement error of the grating harmonics caused by the nonlinear response of the projector is analyzed. Then, the nonlinear of the digital projector is measured by projecting a set of images with different gray levels, and the brightness of these image on the digital projector is measured by optical power meter and the ITF of the projector can be obtained after data processing. Finally, an inverse transformation of ITF is implemened to obtain a corrected non-sinusoidal grating, which is then projected by the projector to obtain a sinusoidal grating on the surface of the measured object. Digital projector measurement experiments show that the average errors of standard panel are 0.71 mm and 0.55 mm before and after calibration, respectively; the average errors of standard block measurement are 0.62 mm and 0.15 mm before and after calibration. The above results show that the proposed method can reduce the measurement error caused by the nonlinear response of the system and improve the measurement accuracy.
-
Key words:
- phase measurement /
- intensity transform function /
- nonlinear /
- calibration
-
表 1 反函数数值解表
Table 1. Numerical solutions of inverse function
归一化的输出功率 归一化的输入灰度 归一化的输出功率 归一化的输入灰度 0 0.082 0.542 0.730 0.042 0.227 0.583 0.756 0.083 0.310 0.625 0.781 0.125 0.373 0.667 0.805 0.167 0.425 0.708 0.828 0.208 0.471 0.750 0.851 0.250 0.512 0.792 0.873 0.292 0.549 0.833 0.894 0.333 0.584 0.875 0.916 0.375 0.616 0.917 0.937 0.417 0.647 0.958 0.957 0.458 0.676 1 0.977 0.500 0.704 表 2 标准平板测量结果
Table 2. Measurement results of standard panel
(mm) 1 2 3 4 5 6 7 8 9 标准 0 20 40 60 80 100 120 140 160 校正前 0.11 18.67 39.16 59.33 79.50 101.02 119.35 138.84 160.10 误差 0.11 1.33 0.84 0.67 0.50 1.02 0.65 1.16 0.1 校正后 -0.29 21.22 39.73 60.08 79.86 101.05 119.17 140.89 160.22 误差 0.29 1.22 0.27 0.08 0.14 1.05 0.83 0.89 0.22 表 3 标准量块测量结果比较
Table 3. Measurement results of standard block
(mm) 项目 校正前结果 校正后结果 面1位置 37.08 36.16 面2位置 25.50 24.11 高度差 11.58 12.05 绝对误差 0.62 0.15 相对误差 5.08% 1.23% -
[1] 牛小兵. 光栅投射三维轮廓测量技术的研究[D]. 天津: 天津大学, 2003. http://www.wanfangdata.com.cn/details/detail.do?_type=degree&id=Y590862NIU X B. 3-D Profilometry based on grating projection[D]. Tianjin: Tianjin University, 2003. (in Chinese) http://www.wanfangdata.com.cn/details/detail.do?_type=degree&id=Y590862 [2] CHEN F, BROWN G M, SONG M. Overview of three-dimensional shape measurement using optical methods[J]. Opt. Eng., 2000, 39(1):10-22. doi: 10.1117/1.602438 [3] 金国藩.激光测量学[M].北京:科学出版社, 1998:798-800.JIN G P. Laser Measurement[M]. Beijing:Science Press, 1998:798-800.(in Chinese) [4] 张万祯. 数字投影结构光三维测量方法研究[D]. 浙江: 浙江大学, 2015. http://cdmd.cnki.com.cn/Article/CDMD-10335-1015418953.htmZHANG W ZH. Structured-light three dimensional measurement method based on digital projector[D]. Zhejiang: Zhejiang University, 2015. (in Chinese) http://cdmd.cnki.com.cn/Article/CDMD-10335-1015418953.htm [5] 马淑贞. 光学三维位相轮廓测量技术研究[D]. 昆明: 昆明理工大学, 2003. http://cdmd.cnki.com.cn/article/cdmd-10674-2003102721.htmMA SH ZH. Studies on a optical three-dimension phase profilometry measurement technology[D]. Kunming: Kunming University, 2003. (in Chinese) http://cdmd.cnki.com.cn/article/cdmd-10674-2003102721.htm [6] 安东, 陈李, 丁一飞, 等.光栅投影相位法系统模型及标定方法[J].中国光学, 2015, 8(1):248-254. http://www.chineseoptics.net.cn/CN/abstract/abstract9270.shtmlAN D, CHEN L, DING Y F, et al.. Optical system model and calibration of grating projection phase method[J]. Chinese Optics, 2015, 8(1):248-254.(in Chinese) http://www.chineseoptics.net.cn/CN/abstract/abstract9270.shtml [7] 李万松. 位相测量轮廓术(PMP)应用研究[D]. 四川: 四川大学, 1999.LI W S. Applied Study on Phase Measuring Profilometry(PMP)[D]. Sichuan: Sichuan University, 1999. (in Chinese) [8] 邵双运, 苏显渝, 等.结构光场的非正弦性对调制度测量轮廓术精度的影响[J].激光杂志, 2004, 25(1):26-28. http://www.opticsjournal.net/Abstract.htm?id=OJ061018000713iPlRoUSHAO SH Y, SU X Y, et al.. The influence of non-sinusoidal signal on the measurement precision of MMP[J]. Laser Journal, 2004, 25(1):26-28.(in Chinese) http://www.opticsjournal.net/Abstract.htm?id=OJ061018000713iPlRoU [9] GUO H W, HE H T, CHEN M Y. Gamma correction for digital fringe projection profilometry[J]. Applied Optics, 2004, 43(14):2906-2914. doi: 10.1364/AO.43.002906 [10] 曹益平. 基于数字微镜的位相测量轮廓术[D]. 四川: 四川大学, 2003. http://cdmd.cnki.com.cn/Article/CDMD-10610-2003107339.htmCAO Y P. Digital Micromirror Device Based Measuring Profilometry[D]. Sichuan: Sichuan University, 2003. (in Chinese) http://cdmd.cnki.com.cn/Article/CDMD-10610-2003107339.htm [11] CAO Y P, et al.. Intensity transfer function of DMD and its application in PMP[J]. Proceedings of SPIE, 2002, 4778:83-88. doi: 10.1117/12.473557 [12] 武迎春, 曹益平, 肖焱山.任意相移最小二乘法迭代的在线三维检测[J].光学精密工程, 2014, 22(5):1347-1353. http://kns.cnki.net/KCMS/detail/detail.aspx?filename=gxjm201405032&dbname=CJFD&dbcode=CJFQWU Y CH, CAO Y P, XIAO Y SH. On-line three-diemensional inspection using randomly phase-shifting fringe based on least-square iteration[J]. Optical and Precision Engineering, 2014.22(5):1347-1353.(in Chinese) http://kns.cnki.net/KCMS/detail/detail.aspx?filename=gxjm201405032&dbname=CJFD&dbcode=CJFQ [13] 肖焱山, 曹益平, 等.基于傅里叶频谱分析的相位测量轮廓术系统Gamma非线性校正方法[J].光学学报, 2012, 32(12):1-6. http://www.opticsjournal.net/abstract.htm?id=OJ121019000067pVsYu2XIAO Y SH, CAO Y P, et al.. Gamma nonlinearity correction based on Fourier spectrum analysis for phase measuring profilometry[J]. Optics Journal, 2012, 32(12):1-6.(in Chinese) http://www.opticsjournal.net/abstract.htm?id=OJ121019000067pVsYu2 [14] 许平. 提高数字光投影傅里叶变换轮廓术精度的方法研究[D]. 四川: 四川大学, 2006. http://cdmd.cnki.com.cn/article/cdmd-10610-2006188119.htmXU P. Study on improving the precision of FTP based on DLP projector[D]. Sichuan: Sichuan Unversity, 2006. (in Chinese) http://cdmd.cnki.com.cn/article/cdmd-10610-2006188119.htm [15] 杨丽霞. 光栅投影三维形貌测量系统的研究[D]. 辽宁: 东北大学, 2012. http://cdmd.cnki.com.cn/Article/CDMD-10145-1014180002.htmYANG L X. Research on the system of grating projection three-dimensional profilometry[D]. Liaoning: Northeastern University, 2012. (in Chinese) http://cdmd.cnki.com.cn/Article/CDMD-10145-1014180002.htm [16] 费东, 苏显渝, 李杰林.PMP中的液晶投影器的性能研究[J].光电工程, 1996, 23(5):18-22. http://www.cqvip.com/QK/60129A/199704/3001015365.htmlFEI D, SU X Y, LI J L. Research on performance of liquid crystal display in phase measuring profilometry[J]. Opto-Electronic Engineering, 1996, 23(5):18-22.(in Chinese) http://www.cqvip.com/QK/60129A/199704/3001015365.html [17] 郝煜栋, 赵洋, 李达成.光栅投影式轮廓测量中两种误差的分析[J].光学学报, 2000, 20(3):376-379. doi: 10.3321/j.issn:0253-2239.2000.03.016HAO Y D, ZHAO Y, LI D CH. Analysis of two errors in grating projection profilometry[J]. Optics Journal, 2000, 20(3):376-379.(in Chinese) doi: 10.3321/j.issn:0253-2239.2000.03.016 [18] 李万松, 苏显渝, 等.非恒定环境光条件下的相位测量剖面术[J].光学学报, 2000, 20(5):617-623. doi: 10.3321/j.issn:0253-2239.2000.05.007LI W S, SU X Y, et al.. Phase-measuring profilometry in non-constant environmental light[J]. Optics Journal, 2000, 20(5):617-623.(in Chinese) doi: 10.3321/j.issn:0253-2239.2000.05.007 -






 下载:
下载: