基于交比不变性的投影仪标定

杨建柏; 赵建; 孙强

doi:10.37188/CO.2020-0111

基于交比不变性的投影仪标定

doi: 10.37188/CO.2020-0111

杨建柏^{1, 2,},
赵建^1, ,,
孙强¹

1.
中国科学院长春光学精密机械与物理研究所，吉林长春 130033
2.
中国科学院大学，北京 100049

基金项目: 国家重点研发计划（No. 2018YFC0308100，No. 2018YFC0307900）；吉林省科技发展计划项目（No. 20190302102GX，No. 20180201048GX）；中国科学院青年创新促进会会员资助项目（No. 2019226）

详细信息

作者简介:
杨建柏（1986—），男，黑龙江海伦人，博士研究生，2009年于中国石油大学获得学士学位，主要从事机器视觉及三维重建方面的研究。E-mail：yang9769@163.com

赵　建（1967—），女，吉林长春人，研究员，博士生导师，主要从事数字图像处理、目标识别与跟踪、视频编解码等方面的研究。E-mail：zhaojian6789@126.com

中图分类号: TB96；TP391
计量
- 文章访问数: 1517
- HTML全文浏览量: 423
- PDF下载量: 137
- 被引次数: 0
出版历程
- 收稿日期: 2020-06-29
- 修回日期: 2020-08-12
- 网络出版日期: 2021-03-05
- 刊出日期: 2021-03-23

Projector calibration based on cross ratio invariance

YANG Jian-bai^{1, 2
,},
ZHAO Jian^{1
, ,},
SUN Qiang¹

1.
Changchun Institute of Optics, Fine Mechanics and Physics, Chinese Academy of Sciences, Changchun 130033, China
2.
University of Chinese Academy of Sciences, Beijing 100049, China

Funds: Supported by National Key Research and Development Project (No. 2018YFC0308100, No. 2018YFC0307900), The Projects of Science Technology Development Plan of Jilin Province (No. 20190302102GX, No. 20180201048GX), Youth Innovation Promotion Association CAS (No. 2019226)

More Information

Corresponding author: zhaojian6789@126.com

摘要

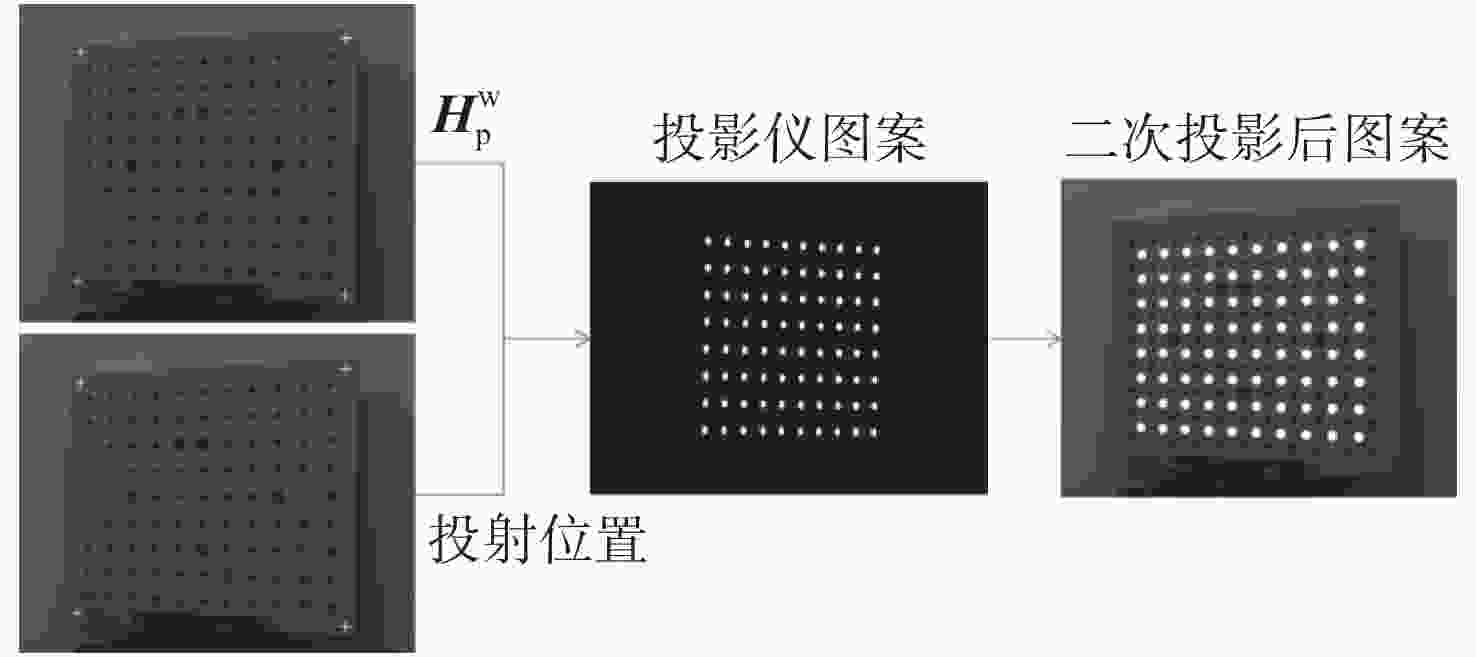
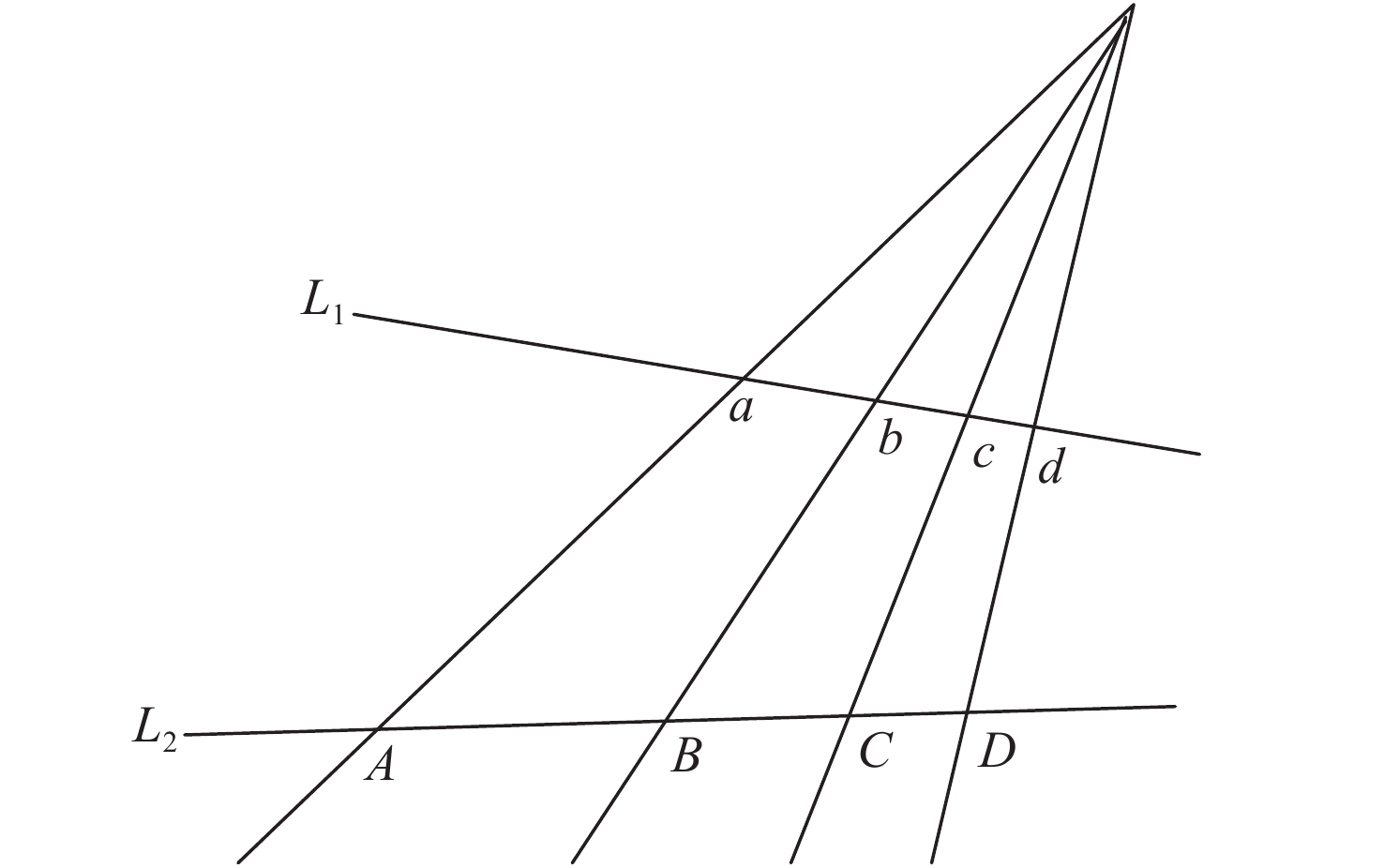
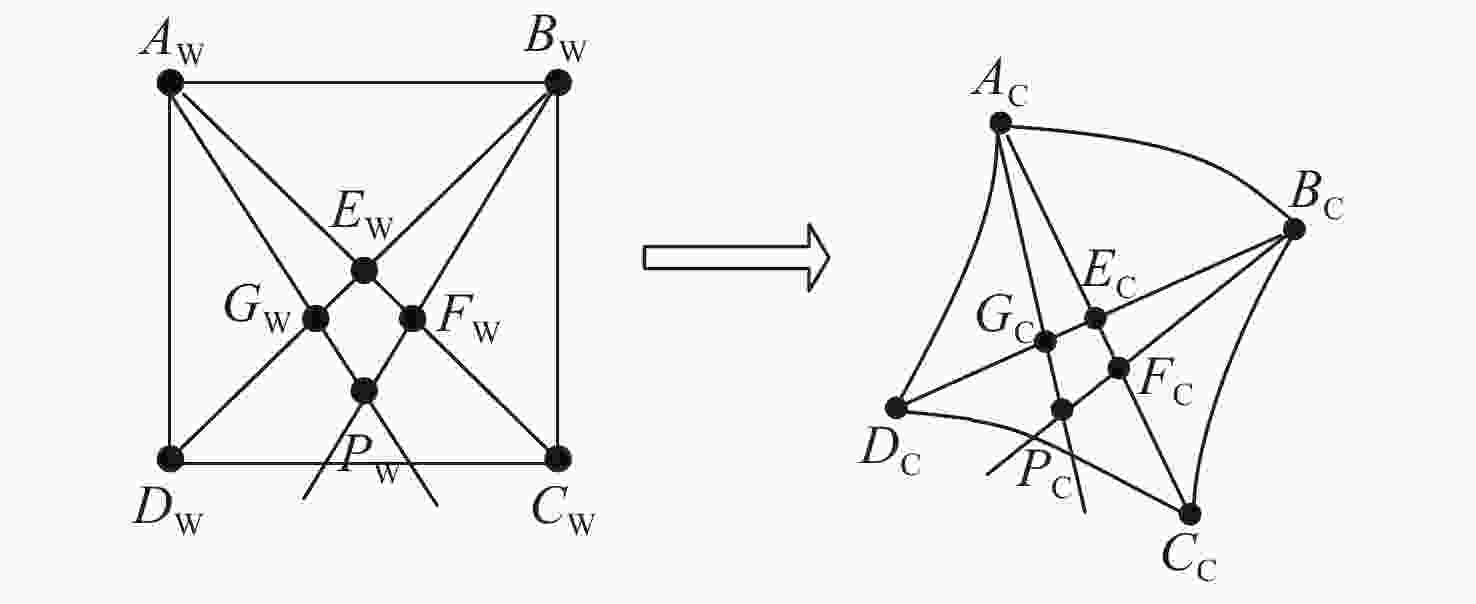
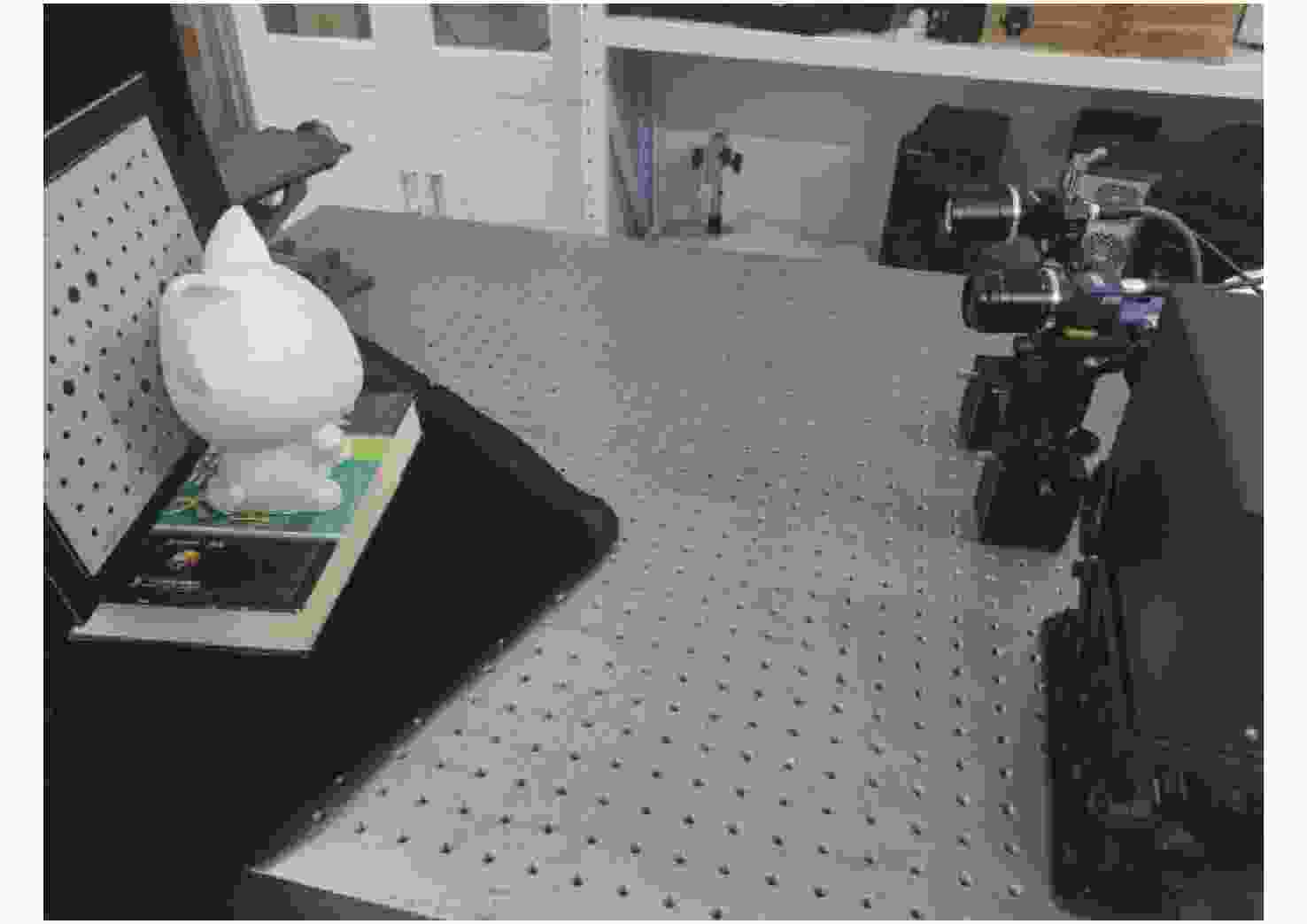
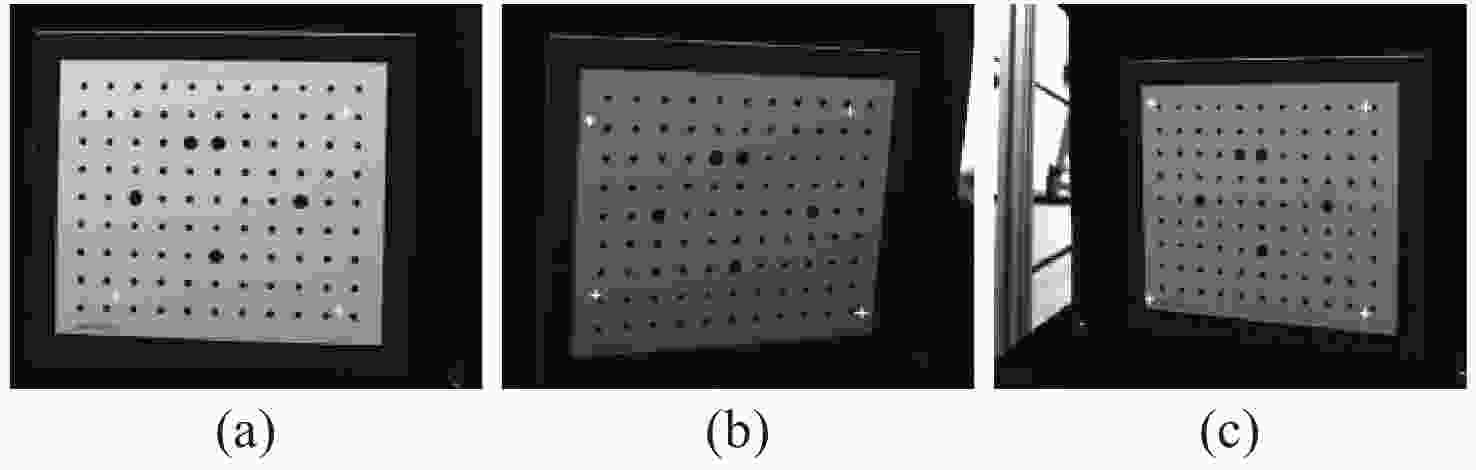
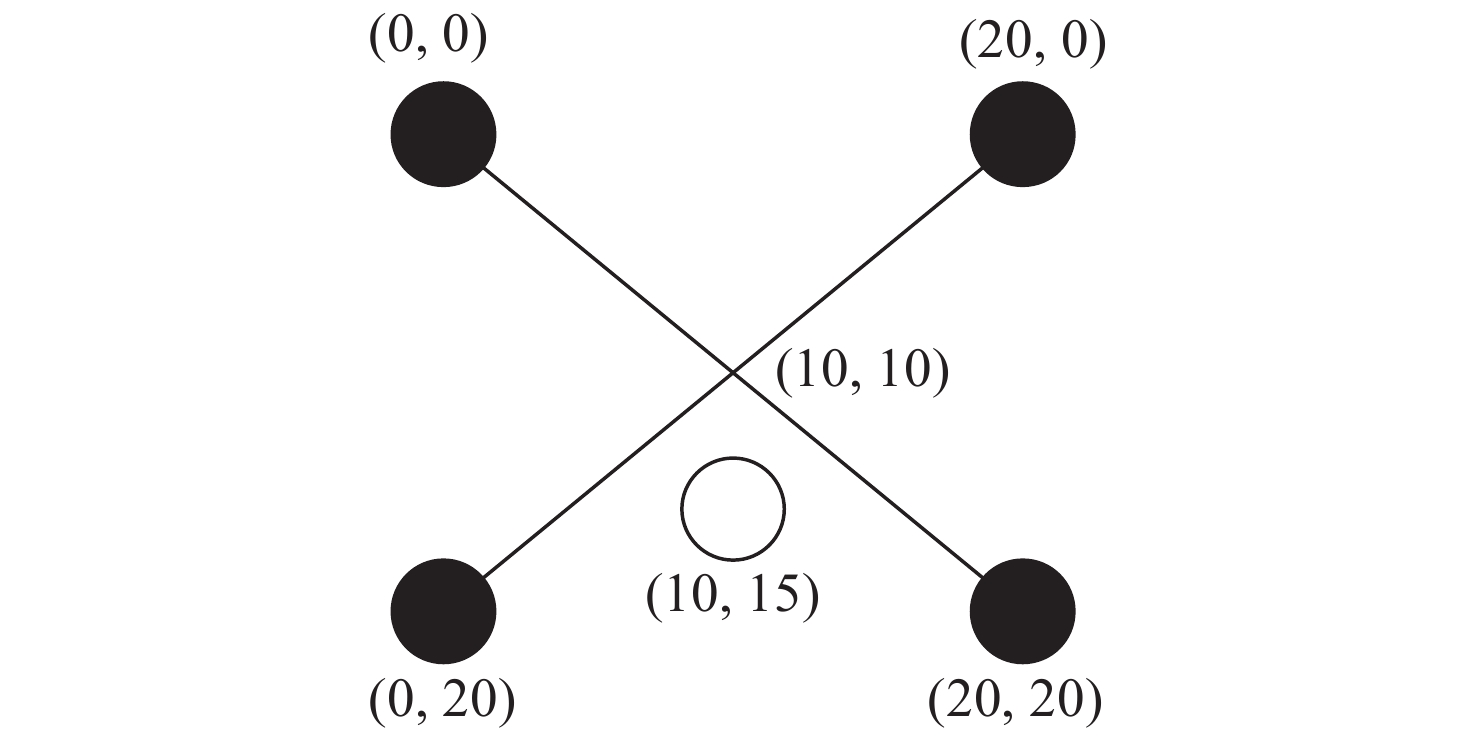
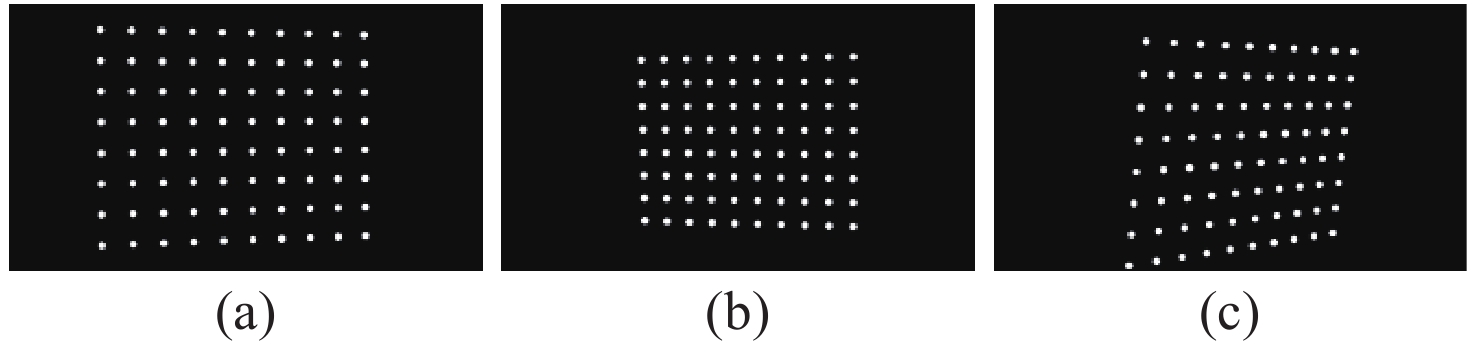
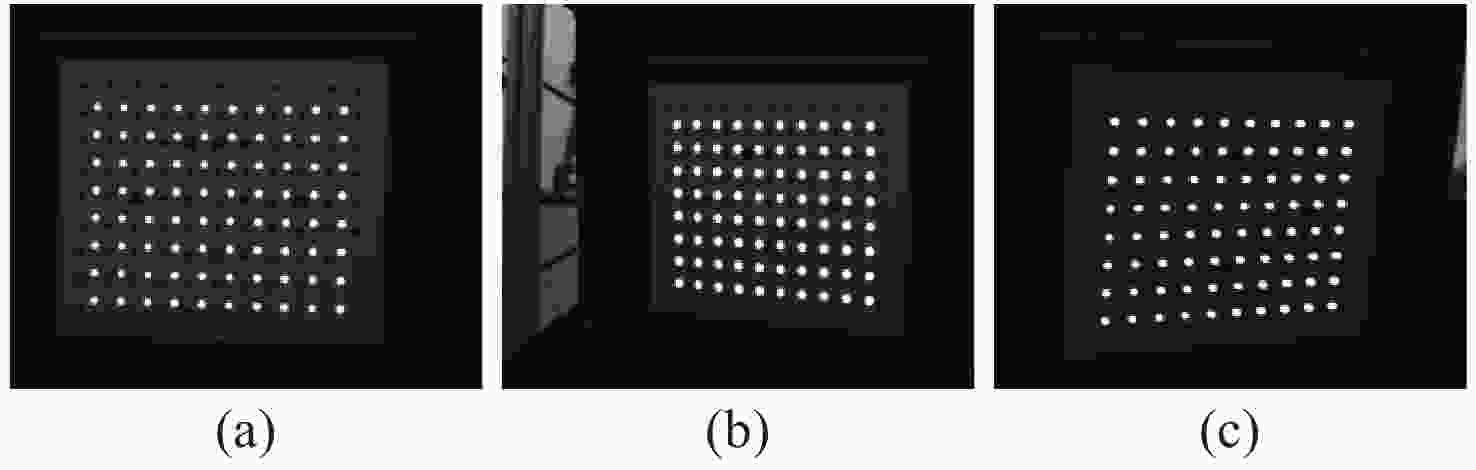
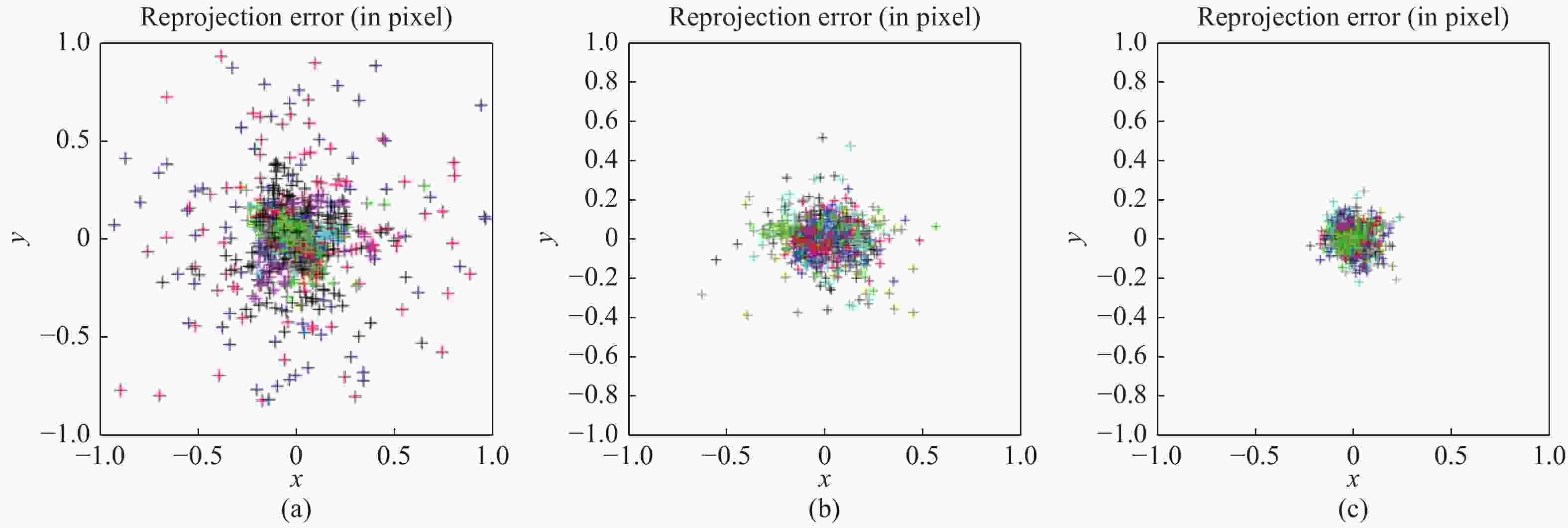
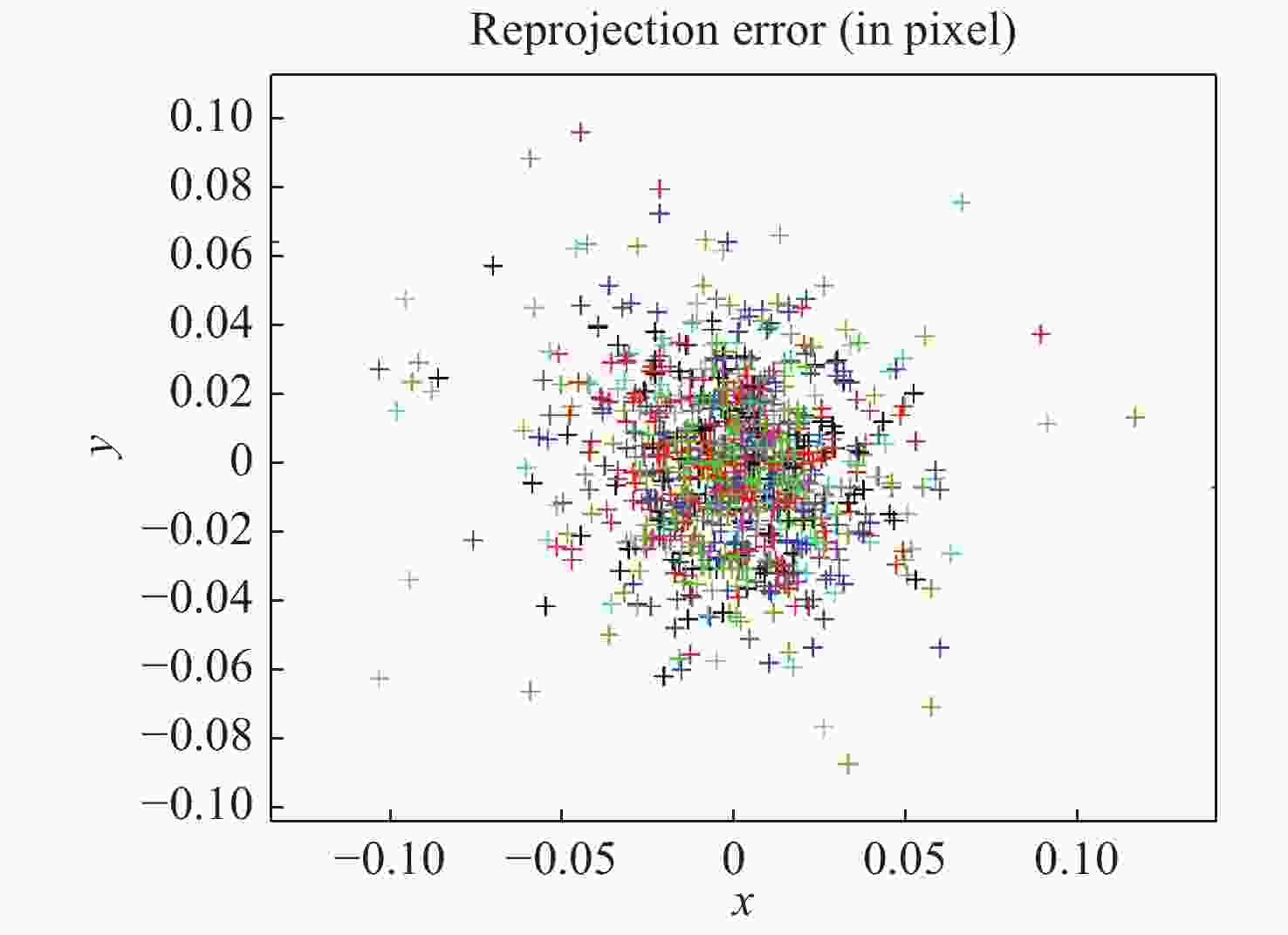
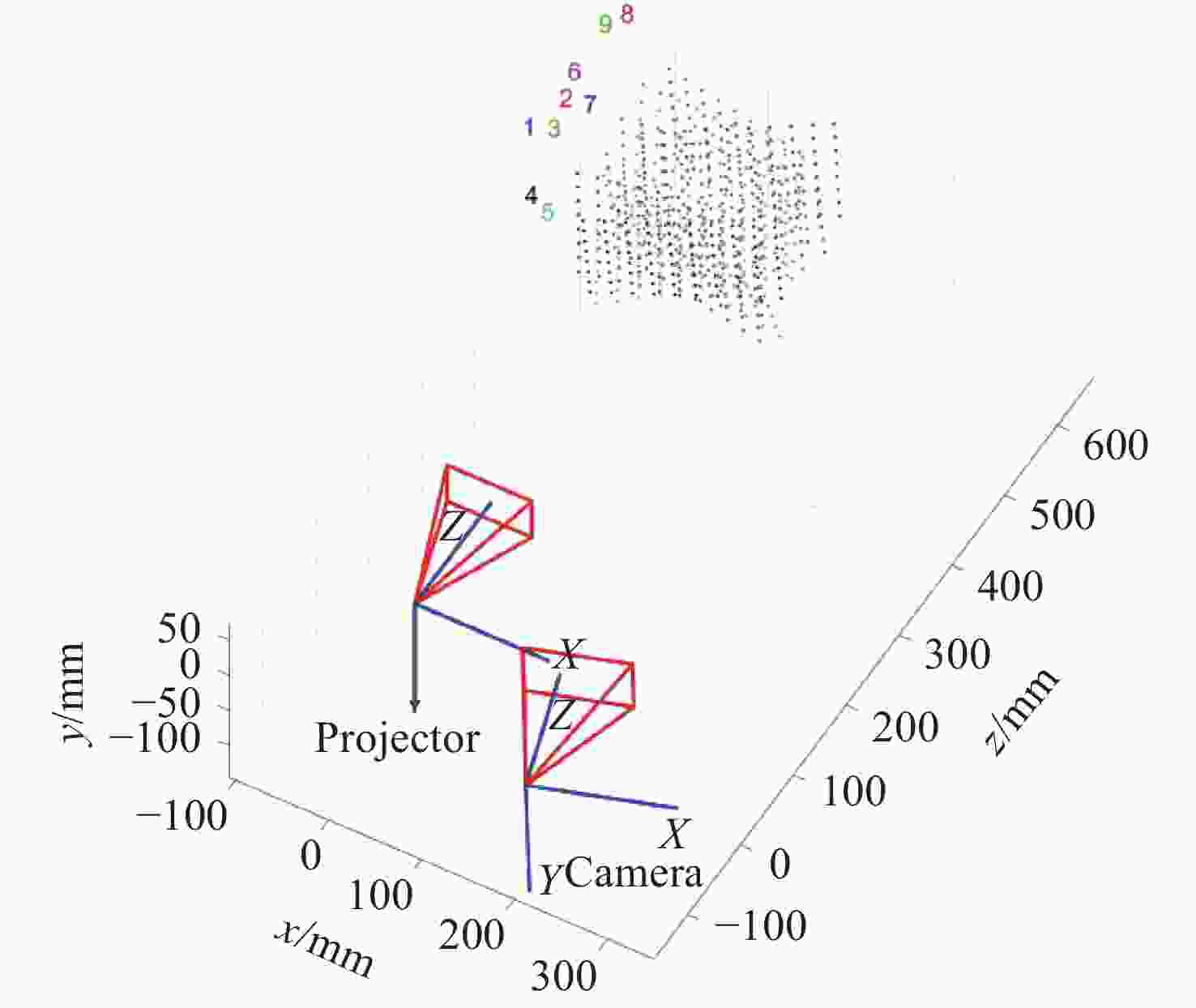
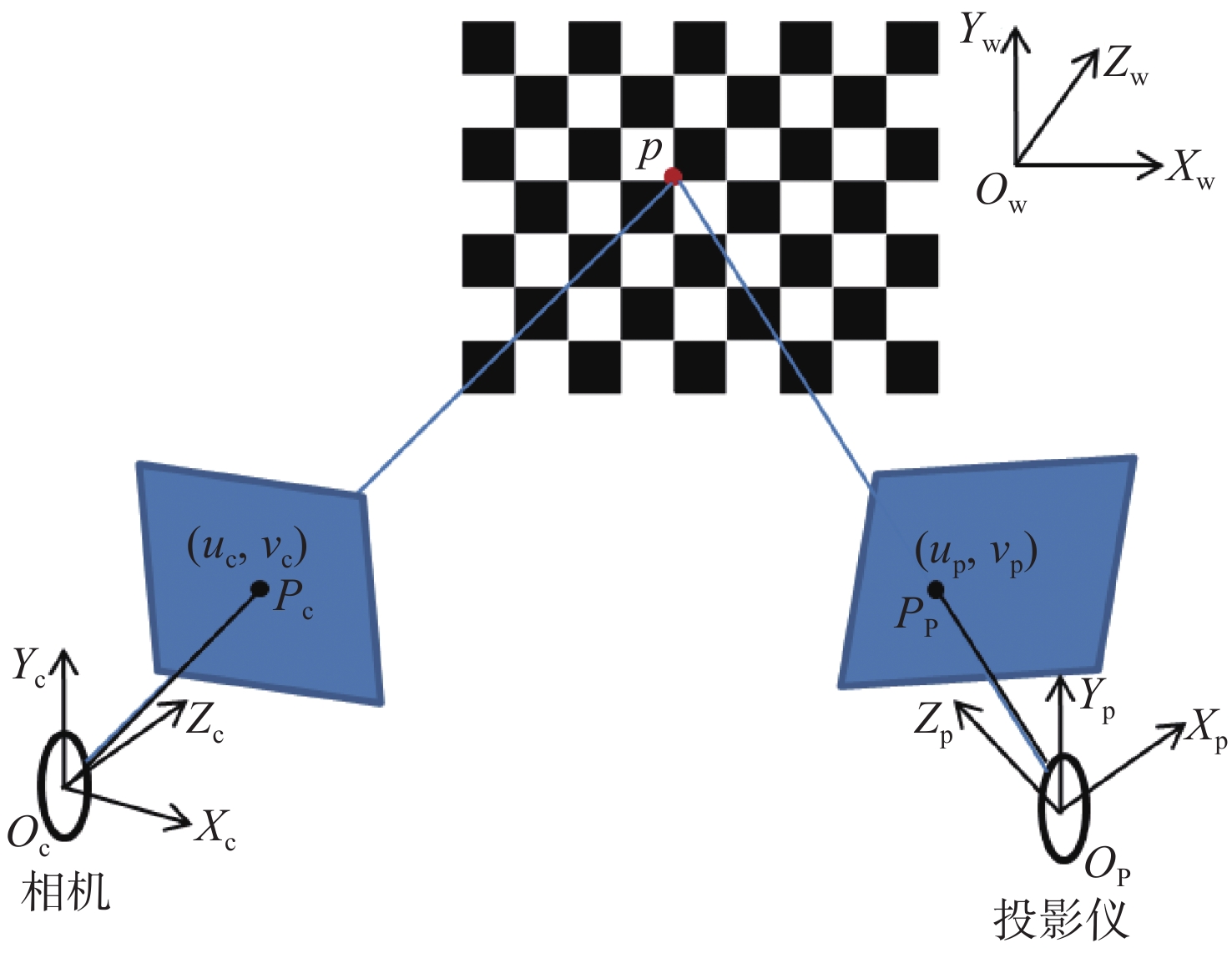
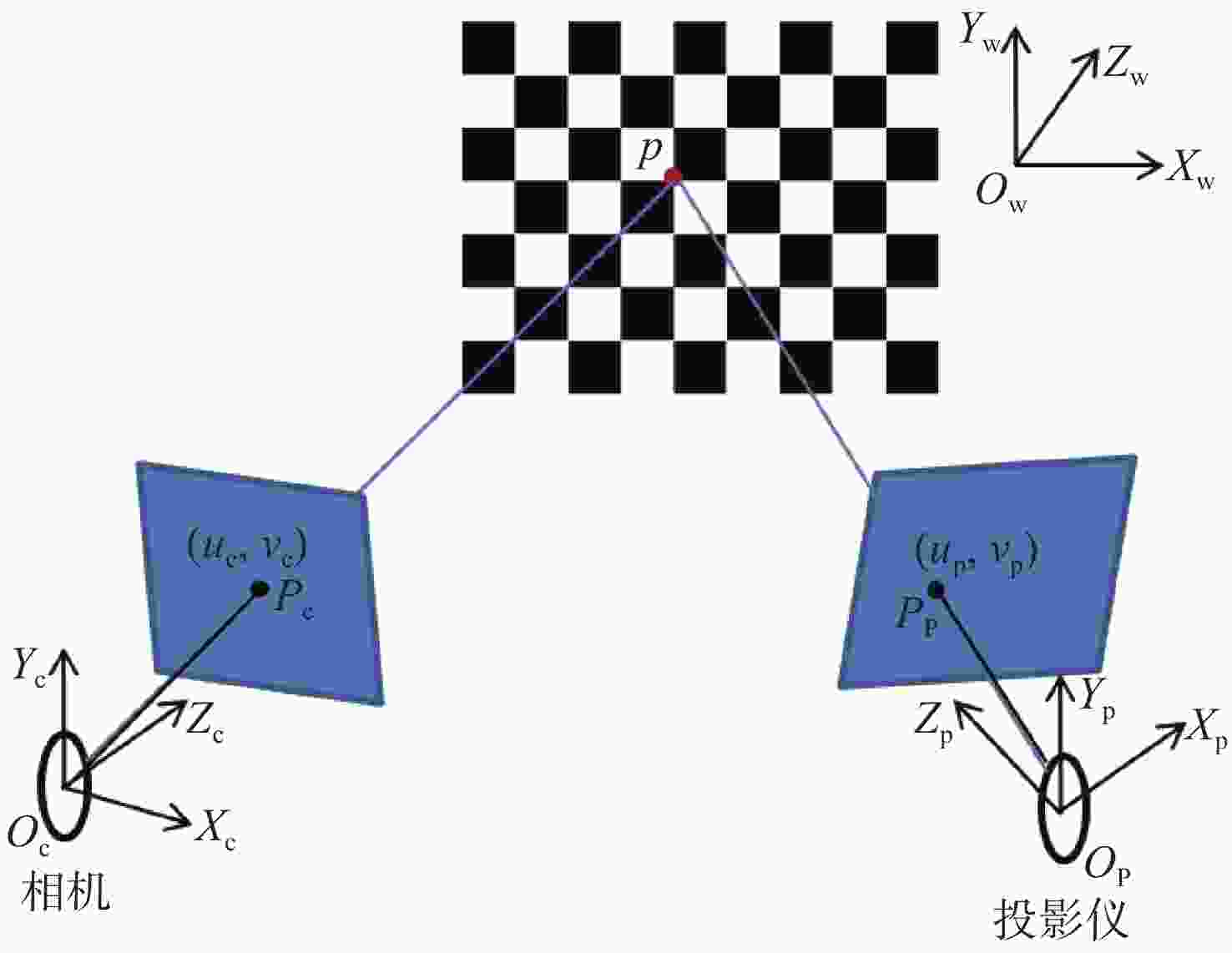
摘要: 提出了一种新的投影仪标定方法以提高数字光栅投影三维测量中投影仪标定的准确性。该方法结合二次投影技术和交比不变性进行投影仪标定。采用二次投影技术解决投射图案与标定板图案互相干扰的问题；采用交比不变性以避免引入相机的标定误差。接着进行了对比实验，以验证所提方法的有效性。选取需要相机参数的传统投影仪标定方法以及根据全局单应性的投影仪标定方法作为对比方法。结果显示，本方法的反投影误差标准差分别从（0.2275, 0.2264）像素和（0.1397, 0.0997）像素降低到（0.0645, 0.0601）像素，反投影误差的最大值分别从1.222像素和0.5617像素降低到0.2421像素。另外，该方法还可同时标定相机，从而获得整个三维测量系统的参数。本文提出的方法可以避免相机标定参数的误差传递，提高投影仪的标定精度。
- 光栅投影 /
- 三维测量 /
- 交比不变性 /
- 投影仪标定
Abstract: In order to improve the accuracy of the projector calibration in 3D shape measurement using digital fringe projection, a new projector calibration method that combines secondary projection technology and cross-ratio invariance is proposed. The secondary projection technology is used to solve the mutual interference between the projection pattern and the calibration pattern, and the cross-ratio invariance method is used to avoid introducing camera calibration error. A comparative experiment is carried out to verify the effectiveness of the proposed method. Compared with the traditional method of projector calibration that requires camera parameters and that using global homography, the RMS values of reprojection error of this method is reduced from (0.2275, 0.2264) and (0.1397, 0.0997) pixels to (0.0645, 0.0601) pixels, and the maximum value of the reprojection error is reduced from 1.222 pixels and 0.5617 pixels to 0.2421 pixels. In addition, this method allows the camera to be simultaneously calibrated during operation, and therefore the parameters of the entire 3D measurement system can be acquired. The above results show that the method proposed in this paper can prevent error propagation of camera calibration parameters and improve the calibration accuracy of a projector.
- digital fringe projection /
- 3D shape measurement /
- cross-ratio invariance /
- projector calibration

HTML全文

图 1 测量系统简化示意图

Figure 1. Schematic diagram of simplified measurement system

下载: 全尺寸图片幻灯片

图 2 基于二次投影的防图案干扰方法

Figure 2. Method for preventing pattern interference based on secondary projection

下载: 全尺寸图片幻灯片

图 3 射影变换示意图

Figure 3. Schematic diagram of projective transformation

下载: 全尺寸图片幻灯片

图 4 交比构成示意图

Figure 4. Schematic diagram of cross-ratio construction

下载: 全尺寸图片幻灯片

图 5 实验系统

Figure 5. Experimental system

下载: 全尺寸图片幻灯片

图 6 第一次投影并采集的图像：（a）姿态1，（b）姿态2，（c）姿态3

Figure 6. The acquired images for the first projection: (a) gesture 1, (b) gesture 2, (c) gesture 3

下载: 全尺寸图片幻灯片

图 7 投射位置示意图

Figure 7. Schematic diagram of the projection position

下载: 全尺寸图片幻灯片

图 8 各姿态投射位置图：（a）姿态1，（b）姿态2，（c）姿态3

Figure 8. Projection positions of each gesture: (a) gesture 1, (b) gesture 2, (c) gesture 3

下载: 全尺寸图片幻灯片

图 9 各姿态生成投射标志点图案：（a）姿态1，（b）姿态2，（c）姿态3

Figure 9. The generated projection point patterns of each gesture: (a) gesture 1, (b) gesture 2, (c) gesture 3

下载: 全尺寸图片幻灯片

图 10 二次投影后采集的标定板图案：（a）姿态1，（b）姿态2，（c）姿态3

Figure 10. Calibration plate patterns acquired after the second projection: (a) gesture 1, (b) gesture 2, (c) gesture 3

下载: 全尺寸图片幻灯片

图 11 几种方法的反投影误差结果。（a）需要相机参数法；（b）全局单应性变换法；（c）本文方法

Figure 11. Reprojection error distribution of different methods. (a) Method requiring camera parameters; (b) the global homography transformation method; (c) the method proposed in this paper

下载: 全尺寸图片幻灯片

图 12 相机标定反投影误差示意图

Figure 12. Schematic diagram of reprojection error in camera calibration

下载: 全尺寸图片幻灯片

图 13 整体系统三维示意图

Figure 13. 3D schematic diagram of the system

下载: 全尺寸图片幻灯片

表 1 标定的投影仪内部参数

Table 1. Calibrated intrinsic parameters of the projector

(pixel)
方法	参数
方法	f_u	f_v	u₀	v₀
需要相机参数标定法	3033.9020	3037.0319	976.0815	546.6816
全局单应性变换法	3040.3878	3042.7892	993.4626	553.0046
本文方法	3060.7594	3059.8479	1006.0491	540.8452

下载: 导出CSV

表 2 标定的投影仪镜头畸变系数

Table 2. Calibrated lens distortion coefficients of the projector

方法	系数
方法	k₁	k₂	p₁	p₂
需要相机参数标定法	0.1102	−0.7058	0.0025	−0.0050
全局单应性变换法	0.0215	−0.4157	0.0033	−0.0014
本文方法	−0.1065	0.0058	0.0011	−0.0007

下载: 导出CSV

表 3 几种方法的反投影误差

Table 3. Reprojection errors of different methods (pixel)

	x轴（MAX）	y轴（MAX）	x轴（STD）	y轴（STD）
需要相机参数标定法	1.222	1.022	0.2275	0.2264
全局单应性变换法	0.5617	0.5130	0.1397	0.0997
本文方法	0.2345	0.2421	0.0645	0.0601

下载: 导出CSV

表 4 相机内部参数和畸变系数标定结果

Table 4. Calibration results of camera intrinsic parameters and distortion coefficients

f_u	f_v	u₀	v₀	k₁	k₂	p₁	p₂
2644.92	2644.11	646.56	508.34	−0.222	0.313	−0.0001	0.0001

下载: 导出CSV

参考文献(19)

[1]	ZHANG S. High-speed 3D shape measurement with structured light methods: a review[J]. Optics and Lasers in Engineering, 2018, 106: 119-131.
[2]	安东, 陈李, 丁一飞, 等. 光栅投影相位法系统模型及标定方法[J]. 中国光学,2015,8(2):248-254. doi: 10.3788/co.20150802.0248 AN D, CHEN L, DING Y F, et al. Optical system model and calibration of grating projection phase method[J]. Chinese Optics, 2015, 8(2): 248-254. (in Chinese) doi: 10.3788/co.20150802.0248
[3]	孟晓亮, 于晓洋, 吴海滨, 等. 基于三维傅里叶变换的胸腹表面测量[J]. 光学精密工程,2018,26(4):778-787. doi: 10.3788/OPE.20182604.0778 MENG X L, YU X Y, WU H B, et al. Measurement of thoraco-abdominal surface using 3D Fourier transform[J]. Optics and Precision Engineering, 2018, 26(4): 778-787. (in Chinese) doi: 10.3788/OPE.20182604.0778
[4]	张旭, 邵双运, 祝祥, 等. 光学三维扫描仪光强传递函数的测量和校正[J]. 中国光学,2018,11(1):123-130. doi: 10.3788/co.20181101.0123 ZHANG X, SHAO SH Y, ZHU X, et al. Measurement and calibration of the intensity transform function of the optical 3D profilometry system[J]. Chinese Optics, 2018, 11(1): 123-130. (in Chinese) doi: 10.3788/co.20181101.0123
[5]	吴坤帅, 魏仲慧, 何昕, 等. 基于笔划三维深度特征的签名识别[J]. 液晶与显示,2019,34(10):1013-1020. doi: 10.3788/YJYXS20193410.1013 WU K SH, WEI ZH H, HE X, et al. Signatures recognition based on strokes 3 D depth feature[J]. Chinese Journal of Liquid Crystals and Displays, 2019, 34(10): 1013-1020. (in Chinese) doi: 10.3788/YJYXS20193410.1013
[6]	马国庆, 刘丽, 于正林, 等. 大型复杂曲面三维形貌测量及应用研究进展[J]. 中国光学,2019,12(2):214-228. doi: 10.3788/co.20191202.0214 MA G Q, LIU L, YU ZH L, et al. Application and development of three-dimensional profile measurement for large and complex surface[J]. Chinese Optics, 2019, 12(2): 214-228. (in Chinese) doi: 10.3788/co.20191202.0214
[7]	任国印, 吕晓琪, 杨楠, 等. 心脏体素化三维模型感兴趣血管交互式显示方法研究[J]. 液晶与显示,2018,33(5):433-442. doi: 10.3788/YJYXS20183305.0433 REN G Y, LV X Q, YANG N, et al. Interactive display methods of vessel of interest within voxelized three-dimensional cardiac model[J]. Chinese Journal of Liquid Crystals and Displays, 2018, 33(5): 433-442. (in Chinese) doi: 10.3788/YJYXS20183305.0433
[8]	ZHANG ZH Y. A flexible new technique for camera calibration[J]. IEEE Transactions on Pattern Analysis and Machine Intelligence, 2000, 22(11): 1330-1334. doi: 10.1109/34.888718
[9]	王谭, 王磊磊, 张卫国, 等. 基于张正友标定法的红外靶标系统[J]. 光学精密工程,2019,27(8):1828-1835. doi: 10.3788/OPE.20192708.1828 WANG T, WANG L L, ZHANG W G, et al. Design of infrared target system with Zhang Zhengyou calibration method[J]. Optics and Precision Engineering, 2019, 27(8): 1828-1835. (in Chinese) doi: 10.3788/OPE.20192708.1828
[10]	赵亚凤, 胡峻峰. 一种双正交消隐点的双目相机标定方法[J]. 液晶与显示,2016,31(10):958-966. doi: 10.3788/YJYXS20163110.0958 ZHAO Y F, HU J F. Binocular self calibration using two pairs of orthogonal vanishing points[J]. Chinese Journal of Liquid Crystals and Displays, 2016, 31(10): 958-966. (in Chinese) doi: 10.3788/YJYXS20163110.0958
[11]	DAVIES E R. 计算机与机器视觉: 理论、算法与实践[M]. 北京: 机械工业出版社, 2013. DAVIES E R. Computer and Machine Vision: Theory, Algorithms, Practicalities[M]. Beijing: China Machine Press, 2013. (in Chinese).
[12]	ZHANG S, HUANG P S. Novel method for structured light system calibration[J]. Optical Engineering, 2006, 45(8): 083601. doi: 10.1117/1.2336196
[13]	LI ZH W, SHI Y SH, WANG C J, et al. Accurate calibration method for a structured light system[J]. Optical Engineering, 2008, 47(5): 053604. doi: 10.1117/1.2931517
[14]	ZHANG W, LI W SH, YU L D, et al. Sub-pixel projector calibration method for fringe projection profilometry[J]. Optics Express, 2017, 25(16): 19158-19169. doi: 10.1364/OE.25.019158
[15]	吴海滨, 于晓洋. 应用市售设备的结构光系统模型及标定[J]. 光学精密工程,2008,16(4):617-623. WU H B, YU X Y. Structured light system model using off-the-shelf components and its calibration[J]. Optics and Precision Engineering, 2008, 16(4): 617-623. (in Chinese)
[16]	AUDET S, OKUTOMI M. A user-friendly method to geometrically calibrate projector-camera systems[C]. 2009 IEEE Computer Society Conference on Computer Vision and Pattern Recognition Workshops, IEEE, 2009: 47-54.
[17]	ANWAR H. Calibrating projector flexibly for a real-time active 3D scanning system[J]. Optik, 2018, 158: 1088-1094. doi: 10.1016/j.ijleo.2018.01.005
[18]	高治华, 王昭, 黄军辉, 等. 基于射影变换的结构光测量系统中投影仪标定方法[J]. 中国激光,2012,39(10):154-161. GAO ZH H, WANG ZH, HUANG J H, et al. Projector calibration method based on projective transformation for structured light measurement system[J]. Chinese Journal of Lasers, 2012, 39(10): 154-161. (in Chinese)
[19]	HARTLEY R, ZISSERMAN A. Multiple View Geometry in Computer Vision[M]. Cambridge: Cambridge University Press, 2003.