-
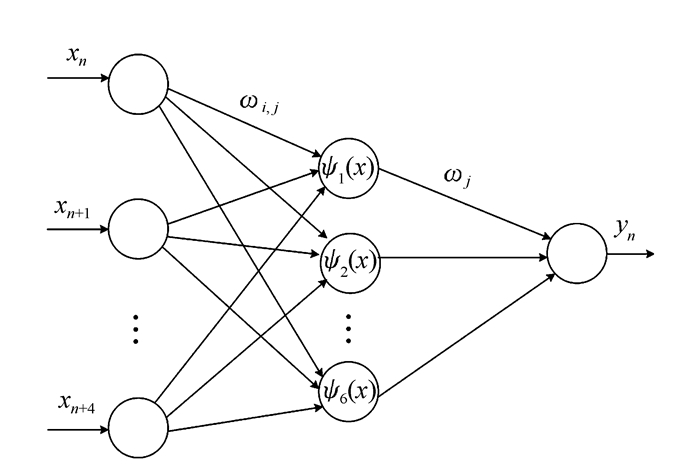
摘要: 为了提高光纤陀螺的测量精度,提出了一种基于小波神经网络的误差补偿方法。首先使用小波分析中的Mallat分解算法提取出陀螺信号中的主趋势项,对其误差余项进行重构。然后将重构信号作为小波神经网络的目标输出,将原始陀螺信号作为训练样本。为了提高小波神经网络的训练速度同时防止其陷入局部极小值,采用增加动量因子和自适应调整学习速率的方法来改进训练方法。训练后建立的神经网络模型对光纤陀螺误差具有良好的估计能力。结果表明,经过小波神经网络方法补偿后,光纤陀螺的输出精度达到了0.019 4°/s,光纤陀螺的测量性能得到了提高。Abstract: In order to improve the measurement accuracy of fiber optic gyroscope, an error compensation method based on wavelet neural network(WNN) is proposed. Firstly, the main trend term in the gyro signal is extracted by the Mallat decomposition algorithm in wavelet analysis, and the error residuals are reconstructed. The reconstructed signal is then used as the target output of the wavelet neural network, and the original gyro signal is used as the training input. In order to improve the training speed of the WNN and prevent it from falling into local minimum values, the method of increasing the momentum factor and adaptively adjusting the learning rate is used. The neural network model established after training has a good ability to estimate the fiber optic gyro error. The final result shows that after the compensation by the WNN method, the output precision of the fiber optic gyroscope reaches 0.0194°/s, which improves the measurement performance of the fiber optic gyroscope.
-
表 1 不同算法的补偿效果
Table 1. Compensation effects for gyroscope by different methods
补偿方法 Kalman算法 软阈值小波 本文方法 运算耗时/s 0.003 1 0.119 7 0.039 1 补偿后精度/
((°)·s-1)0.130 4 0.115 6 0.019 4 -
[1] 徐丽娜, 邓正隆.SINS初始对准中光纤陀螺EMD滤波[J].中国光学, 2015, 8(6):933-941. http://www.chineseoptics.net.cn/CN/abstract/abstract9365.shtmlXU L N, DENG ZH L. EMD filtering of fiber gyro in initial alignment of SINS[J]. Chinese Optics, 2015, 8(6):933-941.(in Chinese) http://www.chineseoptics.net.cn/CN/abstract/abstract9365.shtml [2] 钱华明, 夏全喜, 阙兴涛, 等.基于Kalman滤波MEMS陀螺仪滤波算法[J].哈尔滨工程大学学报, 2010, 31(9):1217-1221. doi: 10.3969/j.issn.1006-7043.2010.09.015QIAN H M, XIA Q X, QU X T, et al.. Algorithm for a MEMS gyroscope based on Kalman filter[J]. Journal of Harbin Engineering University, 2010, 31(9):1217-1221.(in Chinese) doi: 10.3969/j.issn.1006-7043.2010.09.015 [3] 林旻序, 汪永阳, 戴明, 等.线性加速度计在压电陀螺卡尔曼滤波技术中的应用[J].中国光学, 2011, 4(6):600-605. doi: 10.3969/j.issn.2095-1531.2011.06.009LIN M X, WANG Y Y, DAI M, et al.. Application of linear accelerometer to Kalman filter for piezoelectric gyro[J]. Chinese Optics, 2011, 4(6):600-605.(in Chinese) doi: 10.3969/j.issn.2095-1531.2011.06.009 [4] 金靖, 张忠钢, 王峥, 等.基于RBF神经网络的数字闭环光纤陀螺温度误差补偿[J].光学精密工程, 2008, 16(2):235-240. http://d.old.wanfangdata.com.cn/Periodical/gxjmgc200802010JIN J, ZHANG ZH G, WANG ZH, et al.. Temperature error compensation for digital closed-loop fiber optic gyroscope based on RBF neural network[J]. Opt. Precision Eng., 2008, 16(2):235-240.(in Chinese) http://d.old.wanfangdata.com.cn/Periodical/gxjmgc200802010 [5] 徐清雷, 邓正隆, 张传斌.光纤陀螺刻度因子的建模方法[J].光电工程, 2004, 31(12):4-7. doi: 10.3969/j.issn.1003-501X.2004.12.002XU Q L, DENG ZH L, ZHANG CH B. Modeling method for scale factor of fiber optic gyroscope[J]. Opto-Electronic Engineering, 2004, 31(12):4-7.(in Chinese) doi: 10.3969/j.issn.1003-501X.2004.12.002 [6] 李颖, 陈兴林, 宋申民, 等.小波神经网络用于光纤陀螺漂移度误差辨识[J].光学精密工程, 2007, 15(5):773-778. doi: 10.3321/j.issn:1004-924X.2007.05.025LI Y, CHEN X L, SONG SH M, et al.. Application of wavelet neural network for identification of drifts errors in fiber optical gyroscope[J]. Opt. Precision Eng., 2007, 15(5):773-778.(in Chinese) doi: 10.3321/j.issn:1004-924X.2007.05.025 [7] NAVID GHAFFARZADEH. A new wavelet network based method to estimate the lighting-related risk of failure of power system apparatus[J]. Electrical Power and Energy System, 2016, 78:375-384. doi: 10.1016/j.ijepes.2015.11.109 [8] 汤霞清, 程旭伟, 郭礼斌, 等.小波分析和灰色神经网络融合的光纤陀螺误差建模与补偿[J].中国激光, 2012, 39(10):235-240. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=QK201204843711TANG X Q, CHENG X W, GUO L B, et al.. Error modeling and compensation of fiber optic gyro scope based on wavelet analysis and grey neural network[J]. Chinese J. Lasers, 2012, 39(10):235-240.(in Chinese) http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=QK201204843711 [9] ZHANG Q H, BENVENSITE A. Wavelet Networks[J]. IEEE Transactions on Neural Networks, 1992, 3(6):889-898. doi: 10.1109/72.165591 [10] 丁玉美, 阔永红, 高新波.数字信号处理-时域离散随机信号处理[M].西安:西安电子科技大学出版社, 2002.DING Y M, KUO Y H, GAO X B. Digital Signal Processing-discrete Time Domain Random Signal Processing[M]. Xi'an:Xidian University Press, 2002.(in Chinese) [11] 周哲, 庄良杰, 熊正南, 等.基于小波分析的陀螺漂移趋势项提取[J].中国惯性技术学报, 1999, 7(4):58-60, 69. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=QK199900835912ZHOU ZH, ZHUANG L J, XIONG ZH N, et al.. Trend extraction of gyro's drift based on wavelet analysis[J]. Journal of Chinese Inertial Technology, 1999, 7(4):58-60, 69.(in Chinese) http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=QK199900835912 [12] 方琳, 申冲, 陈熙源.基于小波多尺度变换的光纤陀螺振动误差与补偿[J].传感技术学报, 2012, 25(7):902-906. doi: 10.3969/j.issn.1004-1699.2012.07.007FANG L, SHEN CH, CHEN X Y. Error analysis and compensation for fiber optic gyroscope under vibration based on wavelet multi-scale analysis[J]. Chinese Journal of Sensors and Actuators, 2012, 25(7):902-906.(in Chinese) doi: 10.3969/j.issn.1004-1699.2012.07.007 [13] 徐丽娜, 邓正隆.陀螺漂移特性的小波分析[J].中国惯性技术学报, 2001, 9(3):57-60. doi: 10.3969/j.issn.1005-6734.2001.03.012XU L N, DENG ZH L. Wavelet analysis on gyro drift rate[J]. Journal of Chinese Inertial Technology, 2001, 9(3):57-60.(in Chinese) doi: 10.3969/j.issn.1005-6734.2001.03.012 [14] 高宁.陀螺漂移信号的小波去噪及其误差模型的研究[D].天津: 天津大学, 2003. http://cdmd.cnki.com.cn/Article/CDMD-10056-2004075085.htmGAO N. Research on the wavelet denosing and error modeling of gyro drift signal[D]. Tianjin: Tianjin University, 2003.(in Chinese) http://cdmd.cnki.com.cn/Article/CDMD-10056-2004075085.htm [15] 高鹏毅.BP神经网络分类器优化技术研究[D].武汉: 华中科技大学, 2012. http://www.wanfangdata.com.cn/details/detail.do?_type=degree&id=D298043GAO P Y. Study on the optimization of backpropagation neural network classifier[D]. Wuhan: Huazhong University of Science and Technology, 2012.(in Chinese) http://www.wanfangdata.com.cn/details/detail.do?_type=degree&id=D298043 -






 下载:
下载: